Simple as ABC!
 A right angled triangle
A
B
C
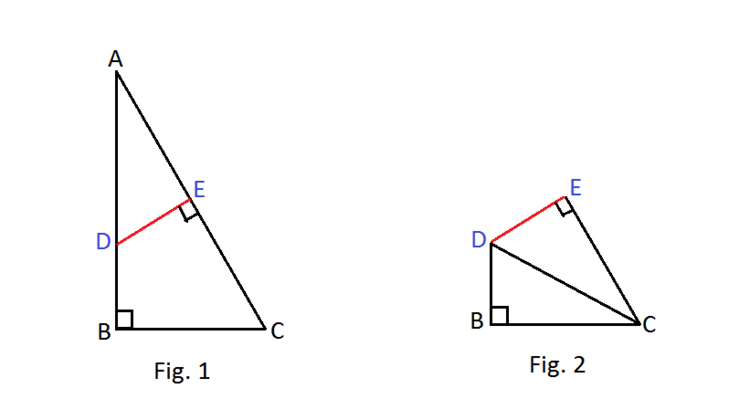
(non-isosceles) was cut from a piece of paper as shown in Fig.1.
A right angled triangle
A
B
C
(non-isosceles) was cut from a piece of paper as shown in Fig.1.
A point E was taken at the mid-point of hypotenuse and the figure was folded along D E such that A coincides with C . As a result, two separate right triangles C B D and C E D were formed. (Fig. 2)
Take A C = h , A B = p , B C = b .
Which of these answer choices is equal to the ratio C D A C ?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
i am totally a idiot
Let's A D = y and A B = x , we already know B C = b . Using Pythagoras theorem we get: y = x 2 + b 2
Now we know that x + y = p , so we have a 2x2 equation system. We solve it and we get:
y = 2 p p 2 + b 2
If we apply Pythagoras theorem to the original triangle we get h 2 = p 2 + b 2
So finally,
A D A C = 2 p h 2 h = h 2 p
Note first that Δ D A E and Δ D C E are congruent right triangles, so
∣ C D ∣ ∣ A C ∣ = ∣ C D ∣ 2 ∗ ∣ E C ∣ = 2 ∗ cos ( ∠ D A E ) = 2 ∗ cos ( ∠ B A C ) = 2 ∗ ∣ A C ∣ ∣ A B ∣ = h 2 p .