A calculus problem by Aaron Jerry Ninan

Calculus Level 5

f ( x ) = sin ⁡ x − a x = 0 \large f(x)=\sin x-ax=0 If the range of real values of a a for which the equation above has at least two real roots is [ α , β ) [\alpha ,\beta ) . Find the value ( β − α ) (\beta -\alpha) to 4 decimal places.


The answer is 1.2172.

Aaron Jerry Ninan by Aaron Jerry Ninan , 20, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

We need to find solutions for s i n ( x ) = a x sin(x)=ax We can see that if a ≥ 1 a \geq 1 then the slope of the line would be too high and it would mean only 1 solution at x=0.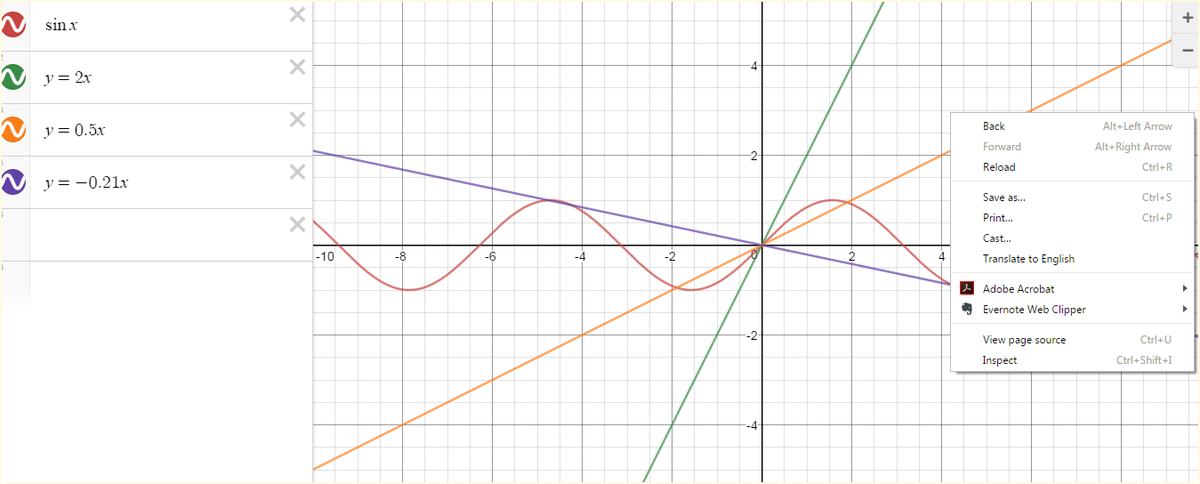 If a < 1 a<1 we have many solutions until we reach a = − 0.2 a=-0.2 when we see that at some value near -0.2, the graph is a tangent to the sin(x) curve and we will have to find exact value of a. Fortunately, it is not that difficult if you write everything in terms of slope and then you get the lower limit of a.

2 Helpful 1 Interesting 1 Brilliant 0 Confused

Desmos it is B)

Sahil Silare - 4 years, 3 months ago

Please add the solution

Vijay Simha - 2 years, 2 months ago

Will add a more detailed solution (especially for the 2nd half) later.

Ajinkya Shivashankar - 4 years, 3 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...