Simple problem (I.Q test)
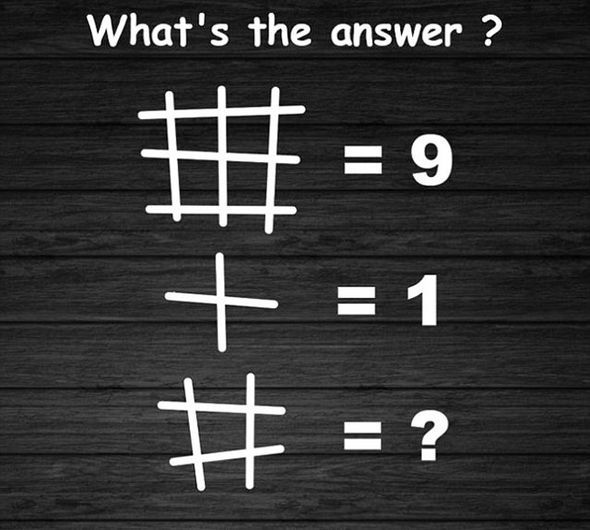 problem
problem
Solve the above problem.
The answer is 4.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
3 2 = 9
1 2 = 1
2 2 = 4
let's solve the sum using BODMAS
In the first figure 3 lines are horizontal and 3 lines are vertical
then let's check which operation makes it = 9:
3+3= 6
3-3=0
3/3 = 1
3x3=9
so multiplication makes it true
even in the second figure:
1 x 1 = 1
1/1=1
1+1=2
1-1=0
but to make the first equation true we keep it multiplication
so in the next figure :
2 x 2 = 4
the problem can be solved using line intersections:
the first diagram has 9 intersections = 9
the second one has 1 intersection = 1
and the last one has four intersections = 4
and exponents too:
1st one has three(3) vertical/horizontal lines so
3 squared or 3 x 3 = 9 so 9
2nd one has one(1) vertical/horizontal lines so
1 squared or 1 x 31 = 1 so 1
3rd one has two(2) vertical/horizontal lines so
2 squared or 2 x 2 = 4
Write how many times the lines cross each other.
Count the intersections and get 4