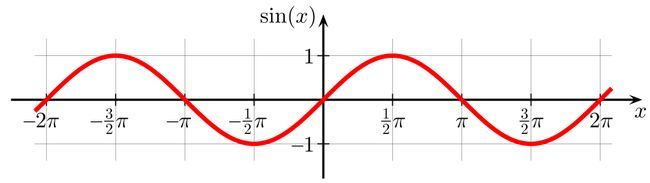
Sine

Well, the problem could have been better but still a nice one.

What is arc length? One can see that it must be a line integral like - $\displaystyle \oint{ds}$ . Now what's $ds$ ? It is the line "fragment" for our arc length i.e. ${(ds)}^{2} = {(dx)}^{2} + {(dy)}^{2}$ , by Pythagoras Theorem.

We can thus change our integral to

$\displaystyle \int_{a}^{b}{\sqrt{1 + {\left(\frac{dy}{dx}\right)}^{2}} dx}$ for some limits $a$ and $b$ .

For our function $y= sin(x)$ over $x = 0$ to $x = 2\pi$ ,

$\displaystyle \text{Arc Length} = \int_{0}^{2\pi}{\sqrt{1 + {cos}^{2}(x)} dx}$

This is of the form of an elliptic integral and more specifically here .

So,

$\displaystyle \text{Arc Length} = 4\sqrt{2} E \left(\frac{1}{\sqrt{2}}\right)$

which becomes

$\displaystyle \frac{8{\pi}^{2} + {\Gamma \left(\frac{1}{4} \right)}^{4}}{\sqrt{2\pi}{\Gamma \left(\frac{1}{4} \right)}^{2}}$