Sinusoidals please

Among the six trigonometric functions, only $\sin x$ and $\cos x$ are sinusoidal.

As x value increases, $\sin x$ is always between -1 and 1. The absolute value of this function is thus between $\inf$ and x, and since x value changes, this value also changes. It is not sinusoidal because it is neither continuous nor periodic.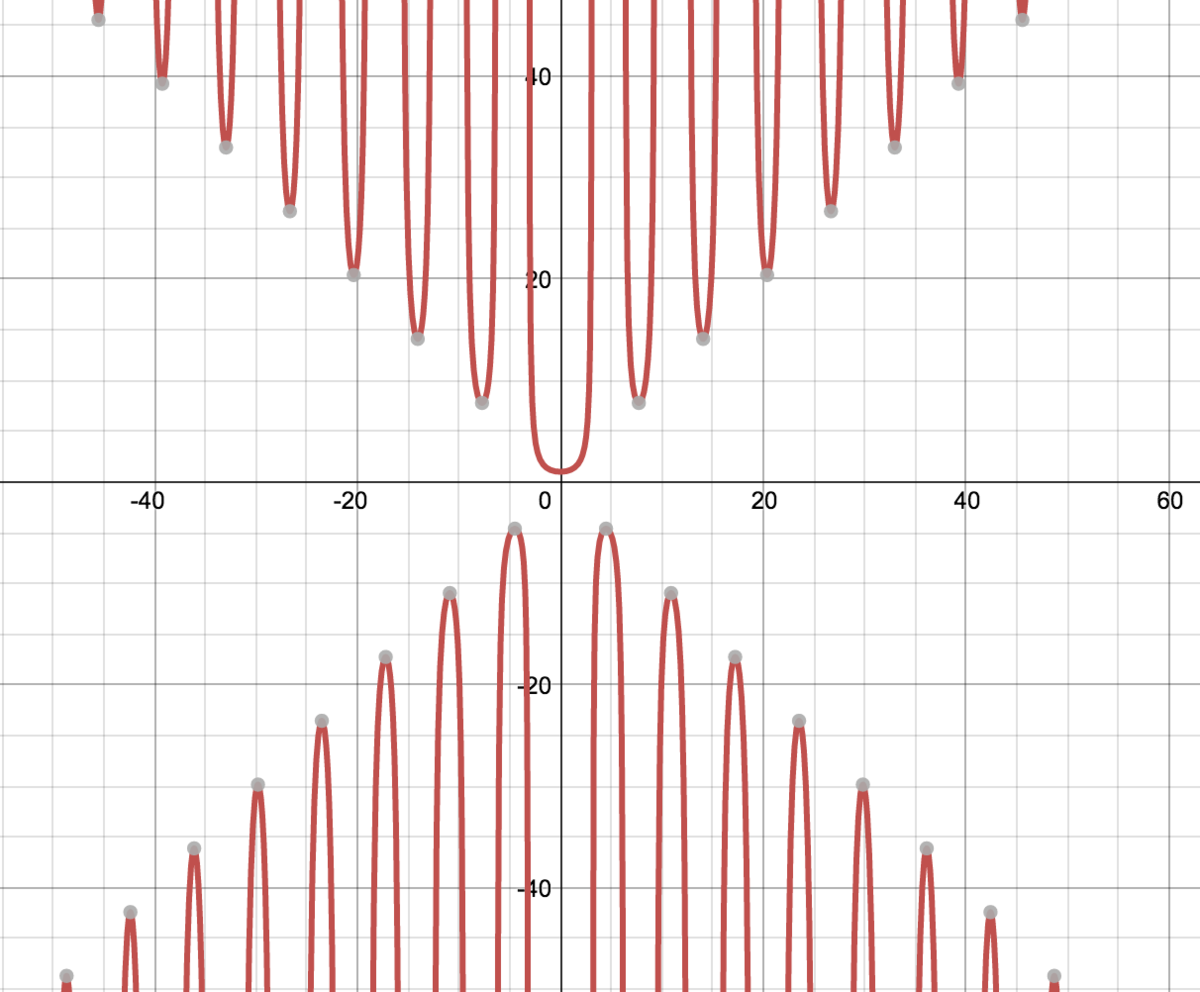

---

Since II) reduces to $\cot x$ , it is not a sinusoidal function because it is not continuous.

---

$\sec 180^\circ = \frac {1}{\cos 180^\circ} = \frac {1}{-1} = -1$ . If $\sin x$ is sinusoidal, $-sin x$ is sinusoidal too. Therefore, III) is sinusoidal. We have $\boxed {2}$ non-sinusoidal functions.