Slime Mold on a Cuboctahedron
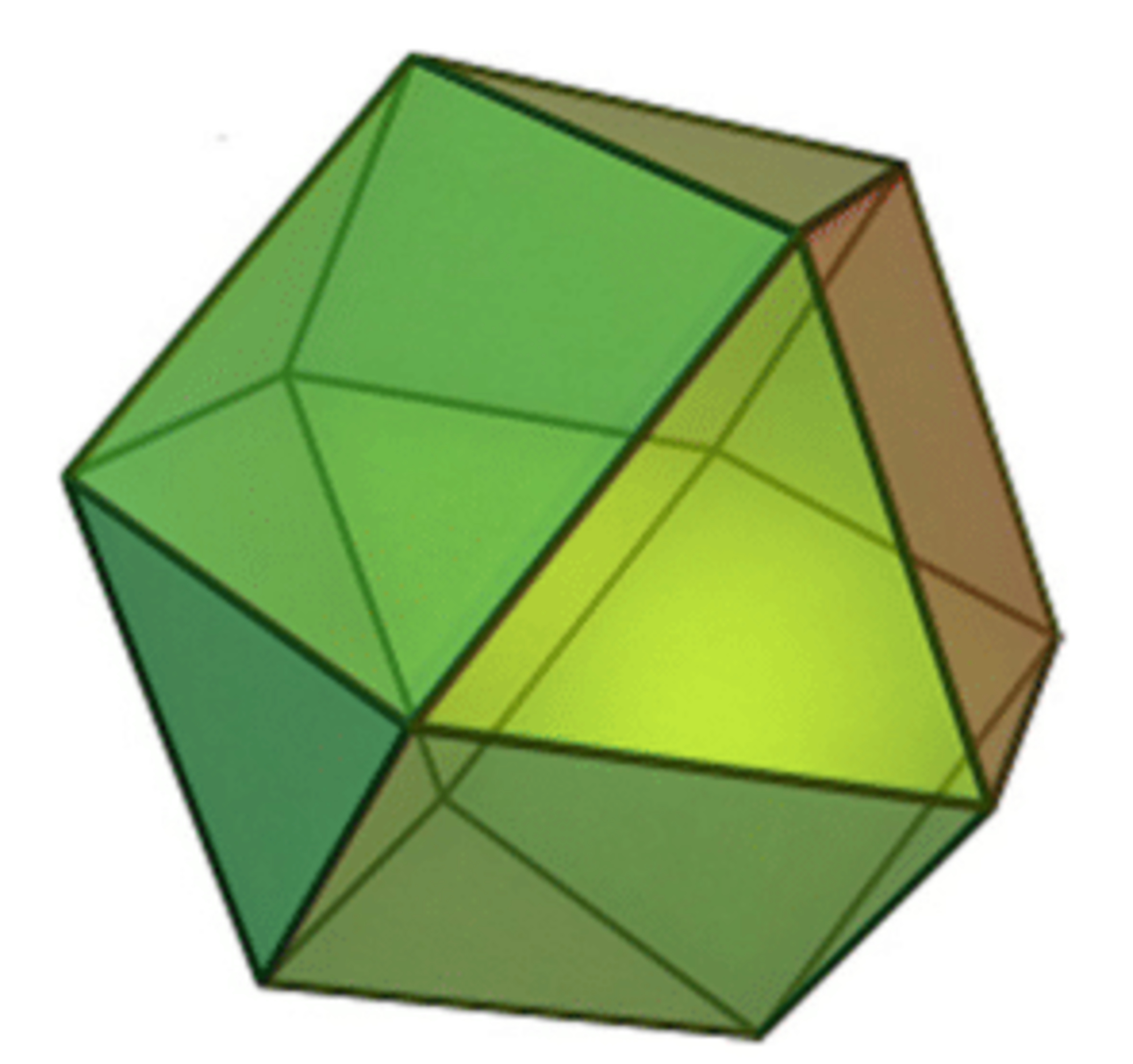
A slime mold, in spite of being really just a collection of single cells, is very good at finding the shortest distance between two points, provided they contain food. Researchers put a bit of oatmeal at two opposing vertices of a cuboctahedron with edge size one. The slime mold's job, and yours, is to find the minimal distance along the surface of the solid between these points. If there is more than one such path, multiply this distance by the number of these paths and report the result to 4 decimal places.
Paths which may be derived from each other by rotation or reflection are considered distinct.
Image credit: Wikimedia POV-Ray .
The answer is 16.3923.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
There are two types of paths, as shown both above.
Distance A D can be obtained easily from the two triangles and the square and is 1 + 3 . The quadrilaterals A B C D and G F E D are congruent. (They contain three unit sides with the angles between them each made up of one 6 0 ∘ and one 9 0 ∘ making them 1 5 0 ∘ ). So the distances A D and D G must be equal.
There are two paths of the type A D and four paths of the type D G , so the final answer is 6 × ( 1 + 3 )