Slow motion for me

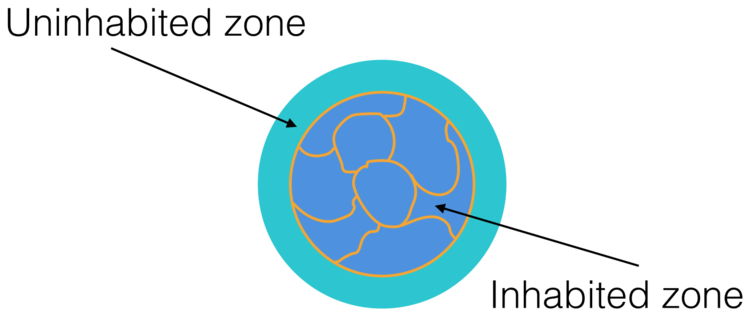A group of bacterium (orange shapes above) sit on a 2d surface that is covered in food with the initial, uniform density $\rho_F$ . We call the inhabited zone (dark blue) the region of the surface that has been reached by the bacteria. As the bacteria grow and divide, the inhabited zone expands into the immediately surrounding uninhabited region, acquiring new food in the process.

As soon as new food is acquired (by expanding the inhabited zone), it is divided equally among all existing bacteria so that the food available to a single bacterium is $c_F = F/N$ , where $F$ is the total amount of uneaten food inside of the inhabited zone.

Finally, the bacteria grow (accumulate mass) according to $\partial_t m = \lambda_\text{max}m\frac{c_F}{\gamma_F + c_F}$ where $\gamma_F$ is the mass of food required to build one new bacterium. When food is highly abundant ( $c_F \gg \gamma_F$ ), the bacteria accumulate mass at the exponential rate $\lambda_\text{max}$ , but as food becomes scarce, they slow down.

Suppose the colony starts with one newly birthed bacteria (of mass $m_0$ ), what is the mass of the bacterial colony after 1,000 hrs (in terms of $m_0$ )?

Assumptions and Details

• Individual bacterium start with mass $m_0$ (and area $\delta_A$ ), and divide into two when they've grown to $2m_0$ (and area $2\delta_A$ ).
• The inhabited zone expands by the growing bacteria inside it pushing each other outward from the center.
• $\lambda_\text{max} = 1\text{ hr}^{-1}$
• $\gamma_F = 0.8\times 10^{-15}$ g
• The density of food in the uninhabited zone is $\rho_F = 1\times 10^{-15}\text{ g}/\delta_A$ .