Solid Square Moment
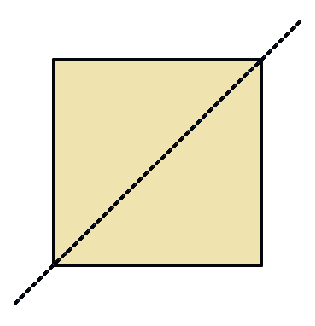
Consider a solid square with side length L , total mass M , and uniform mass density as a function of the area.
Determine the object's moment of inertia about an axis lying in the plane of the square and coinciding with the square's diagonal.
If its value can be represented as α M L 2 , determine the value of α .
For a similar but easier problem, try this .
The answer is 12.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Consider an axis of rotation along one of its lengths. Consider an strip of width d x at a distance x from the axis, it's moment of inertia would be d I = d m ⋅ x 2 = L M d x ⋅ x 2
Thus I = M L 2 / 3 , by integrating the above expression between 0 and L .
Using the perpendicular axis theorem, the moment of inertia about the corner = 2 I . Applying parallel axis theorem we get, 2 I = I C O M + 2 M L 2 I C O M = 2 3 M l 2 − 2 M L 2 = 6 M L 2
Applying perpendicular axis theorem about the center of mass wraps the process, we get 2 I diagonal = I C O M I diagonal = 1 2 M L 2
Edit: Since the body is a square I have used the fact that I x = I y = I and I x + I y = 2 I .
Kindly change a line in the question,uniform mass density as a function of area,it's ambiguous and may lead to reporting
How is it ambiguous?
Let the lower left corner be the origin O and upper left corner and centre of the square be P and Q respectively. Finding the moment of inertia I of the square is equal to 4 × the moment of inertia of △ O P Q . Let the mass per area be λ . Then we have:
I = 4 ∫ 0 2 L ∫ 0 x λ y 2 d y d x = 4 ⋅ L 2 M ∫ 0 2 L ∫ 0 x y 2 d y d x = L 2 4 M ∫ 0 2 L 3 x 3 d x = 3 L 2 4 M 4 x 4 ∣ ∣ ∣ ∣ 0 2 L = 3 L 2 M ( 2 L ) 4 = 1 2 M L 2 Note that λ = L 2 M
⟹ α = 1 2