So Fierce

Geometry Level 4

$\large \sqrt{b^2-10\sqrt2 b + 51} + \sqrt{c^2-2\sqrt{14} c + 30} \leq 5$

Let the $b$ and $c$ denote the base and height of a right triangle $ABC$ , and that they satisfy the constraint above. Let the area of the triangle $ABC$ be denoted as $D$ , find the value of $D^2$ .

The answer is 175.

1 solution

Gabi Dobre
Dec 19, 2015

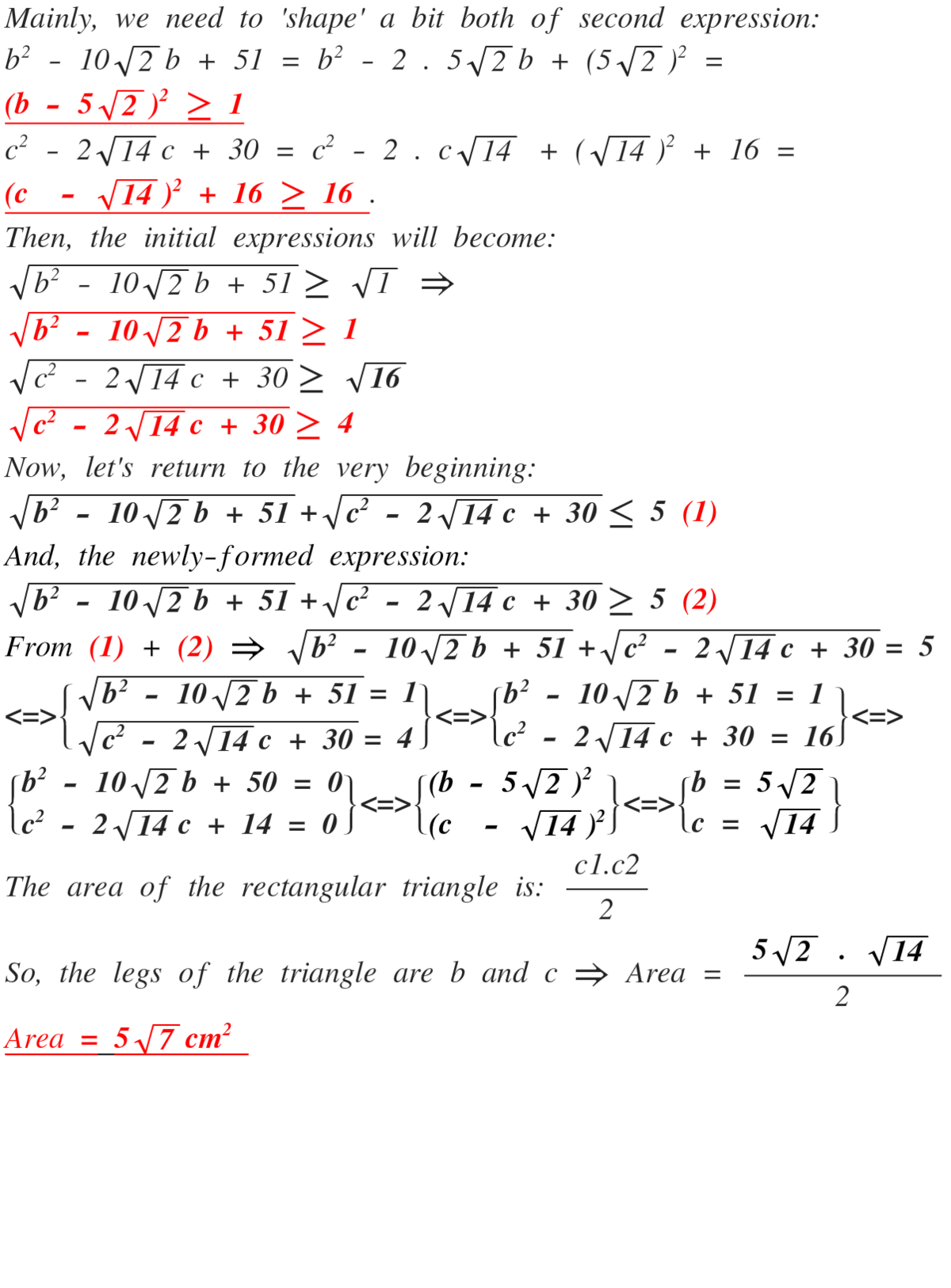and if we square it the final result is 175

Nice solution! I think after the first two lines of $\underline{inequalities}$ it is sufficiently clear that the only values of b and c are $b = 5\sqrt{2}$ and $c = \sqrt{14}$ . This means that $\sqrt{b^{2}-10\sqrt{2}b+51}+\sqrt{c^{2}-2\sqrt{14}c+30}=5$

Chang Jia Geng - 5 years, 5 months ago

So Fierce

The answer is 175.

1 solution

0 pending reports