Moving Particles

Classical Mechanics Level 2

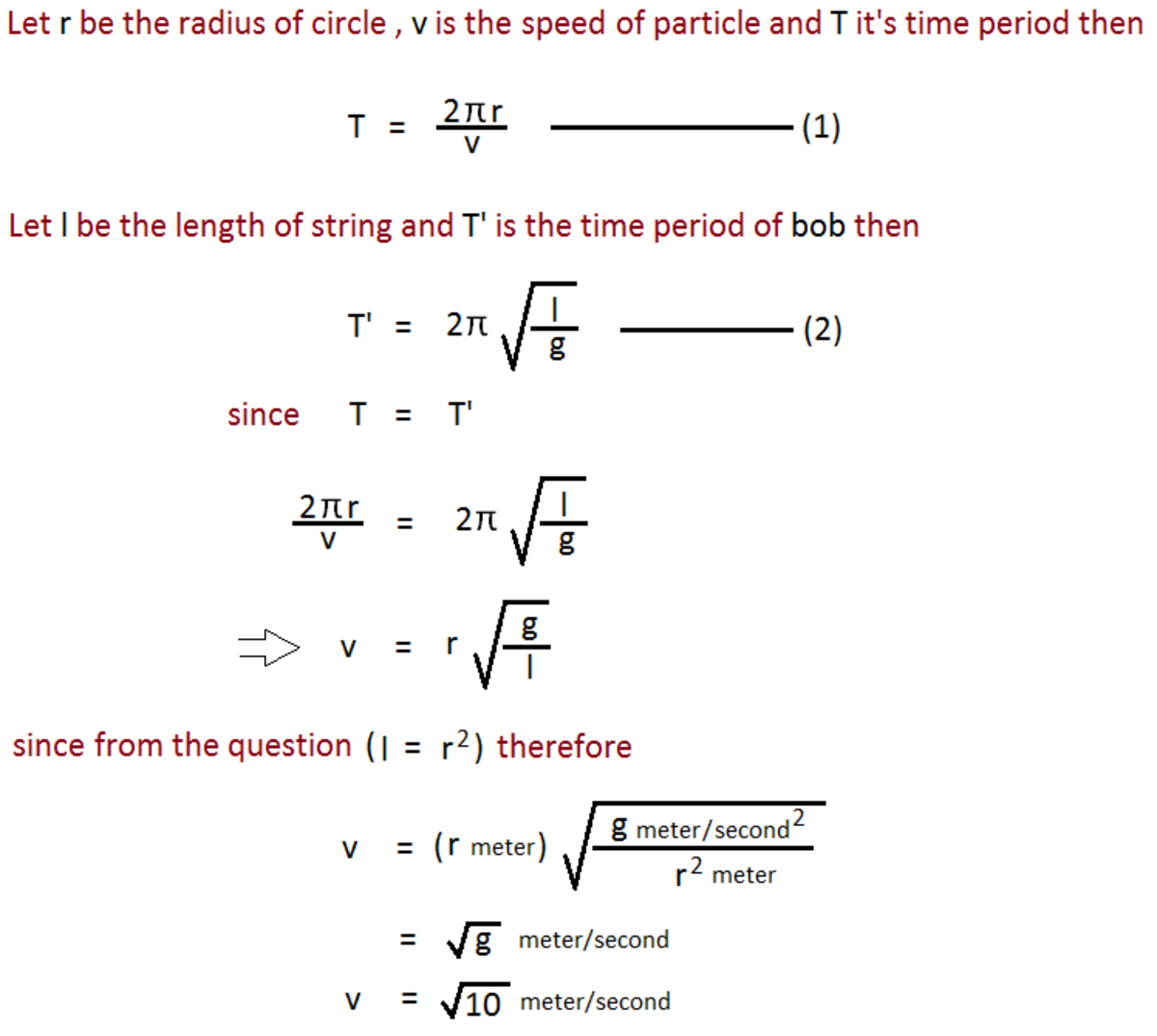
In the figure there are two particles one executing simple harmonic motion and other executing uniform circular motion, both have same time period. If the length of string is equal to the square of radius of circle then find the speed of particle which executing uniform circular motion.

Details

  1. g = 10 ms − 2 g = 10 \text { ms}^{-2}
10 \sqrt{10} 8 \sqrt{8} 5 \sqrt{5} 11 \sqrt{11}

Rahul Paswan by Rahul Paswan , 26, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Rahul Paswan
Jan 4, 2015

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Ujjwal Rane
Jan 29, 2015

Since frequencies of the uniform circular motion (UCM) and the simple harmonic motion (SHM) are same, the angular frequencies will also be the same.

ω U C M = ω S H M \omega_{UCM} = \omega_{SHM}

V r = g L = r 2 \frac{V}{r} = \sqrt{\frac{g}{L=r^2}}

V = g = 10 V = \sqrt{g}=\sqrt{10} . . . cancelling r from both sides

1 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...