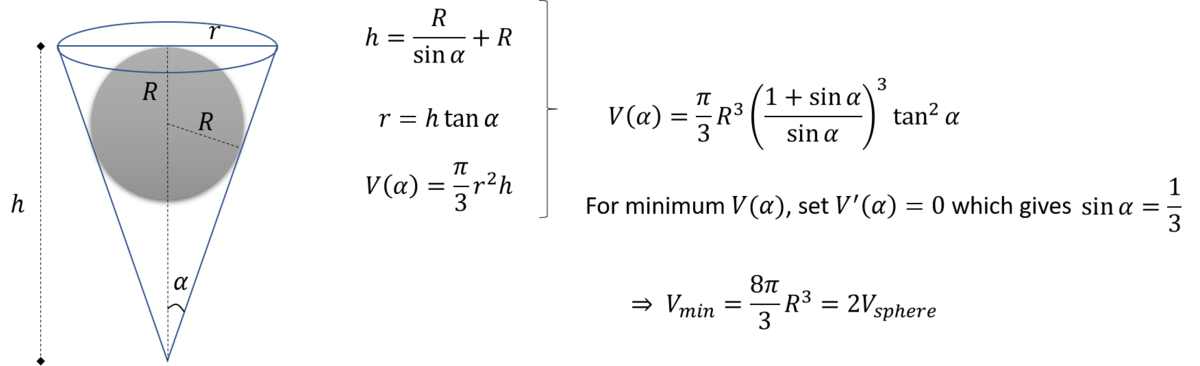
Sphere in a cone?

Geometry Level 3

What is the minimum volume of a cone needed to fully contain a sphere? Report your answer as a ratio of volume of cone to that of sphere.

V c o n e / V s p h e r e {V_{cone}} / {V_{sphere}} = ?


The answer is 2.

Krishnaraj Sambath by Krishnaraj Sambath , 35, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

4 Helpful 1 Interesting 6 Brilliant 0 Confused
David Vreken
Feb 25, 2019

Let R R be the radius of the sphere tangent to the cone, r r be the radius of the cone, and h h be the height of the cone.

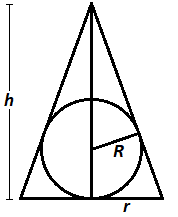
Then by similar triangles, R h − R = r r 2 + h 2 \frac{R}{h - R} = \frac{r}{\sqrt{r^2 + h^2}} , which solves to r 2 = R 2 h 2 h 2 − 2 R h r^2 = \frac{R^2h^2}{h^2 - 2Rh} , which means the volume of the cone is V c o n e = 1 3 π R 2 h 3 h 2 − 2 R h V_{cone} = \frac{1}{3}\pi \frac{R^2h^3}{h^2 - 2Rh} .

For the minimum V c o n e V_{cone} , the derivative V c o n e ′ = 0 V_{cone}' = 0 , which solves to h = 4 R h = 4R .

Therefore, r 2 = 2 R 2 r^2 = 2R^2 , and V c o n e = 8 3 π R 3 = 2 V s p h e r e V_{cone} = \frac{8}{3}\pi R^3 = 2V_{sphere} , so V c o n e V s p h e r e = 2 \frac{V_{cone}}{V_{sphere}} = \boxed{2} .

1 Helpful 0 Interesting 5 Brilliant 0 Confused

This volume function was much easier to minimize than mine!

On the left hand side of your first equation, you want a big R R in the denominator, not a small r r . R h − R = r r 2 + h 2 \frac{R}{h-R} = \frac {r}{\sqrt {r^2+h^2}}

Matthew Feig - 2 years, 3 months ago

Log in to reply

Thanks! I edited it.

David Vreken - 2 years, 3 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...