Spider on Artsy Lampshade

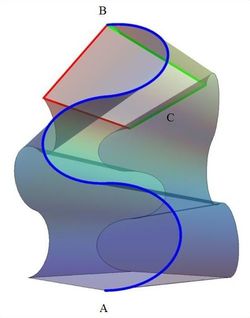A spider at bottom corner $A$ of an artistic lampshade spots Not-So-Brilli-the-Ant at top corner $B$ . Not-So-Brilli-the-Ant panics and starts fleeing counter-clockwise at the top rim of the lampshade, along the green lines. The clever spider, moving twice as fast as Not-So-Brilli-the-Ant, takes the shortest distance on the surface of the lampshade to intercept point $C$ , catches him, wraps him up in silk, and throws him into his food locker.

Here's another view of the lampshade from top down

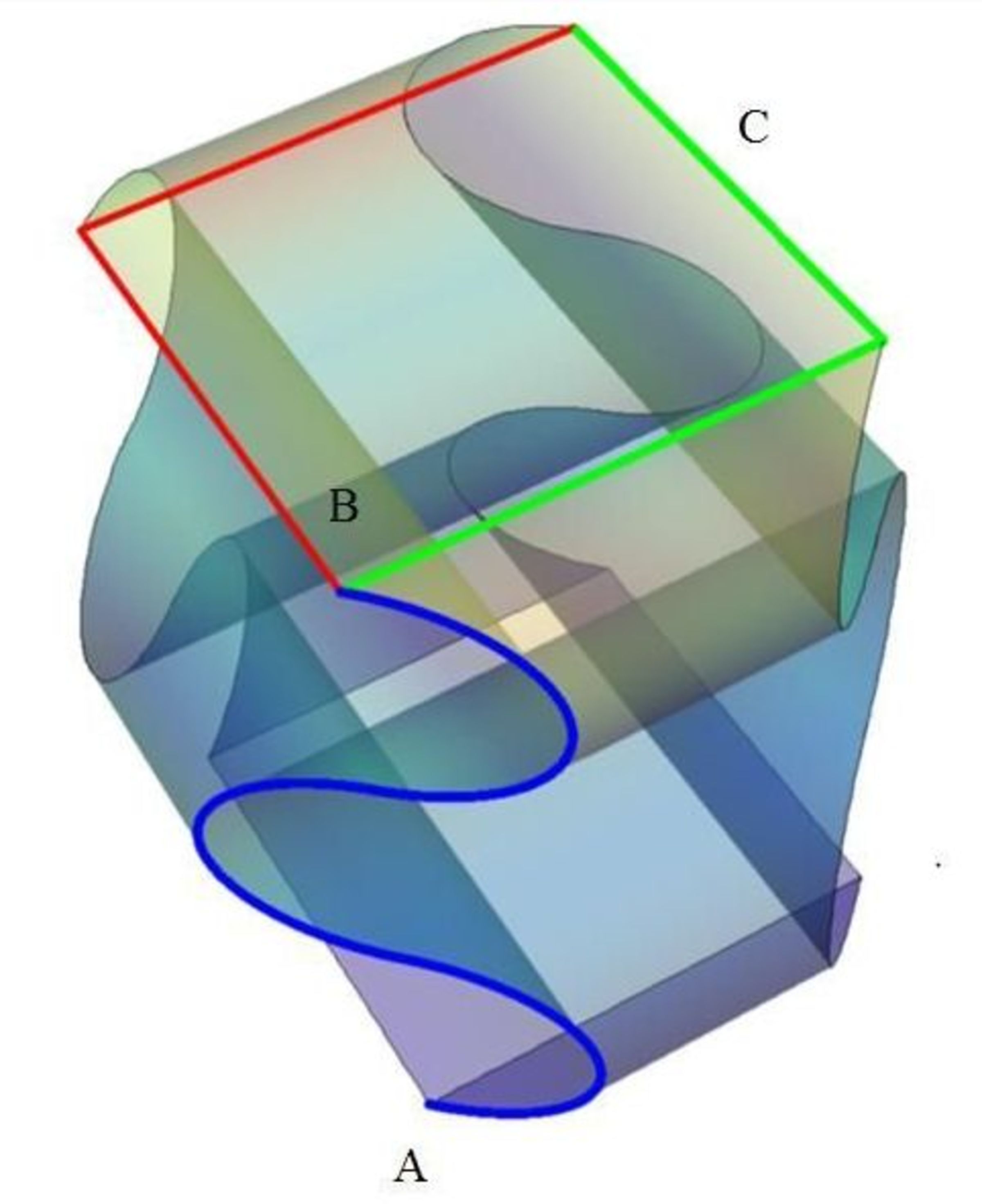
The top and bottom rims of the lampshade are perfect squares with $40$ $cm$ sides. The lampshade is $60$ $cm$ tall from bottom square rim to top square rim. The cross-section profile, orthgonal projections on $xz$ or $yz$ planes, as seen either from the right side or the left side of the spider, is made up of $3$ identical semi-circles stacked vertically. The lampshade is rotationally symmetric, i.e., it looks the same when rotated $180$ degrees about a central vertical axis.

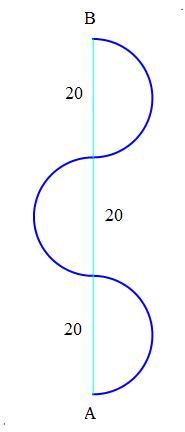
How far did the spider travel from point $A$ to point $C$ ?
If $L$ is the path length on the surface in $cm$ , find $\left\lfloor 10L \right\rfloor$

Okay, you don't need to use calculus for this.