Spin The Beaker

A beaker is filled with water and is being rotated about its vertical axis.
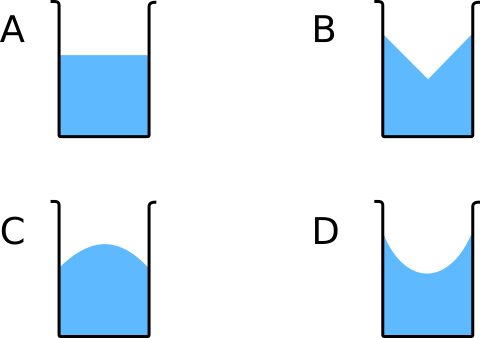
What will the surface of the water look like?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
We can carry out this experiment in real life and see that the shape of the surface of water is very similar to the shape shown in D. We can also find the shape of the surface analytically.
Consider a small cylinder in the radial direction, which is at distance r from the center of the beaker. Its area of cross section is A , and its length is d r . The mass of the cylinder is d m , and its density is ρ .
Since this cylinder is moving in a circle of radius r , the net force on it in the radial direction is d m ω 2 r towards the center.
d F d F d P d r d P = d m ω 2 r = ρ A d r ω 2 r = A d F = ρ ω 2 r d r = ρ ω 2 r
On integrating this equation, we get P = P c e n t e r + 2 ρ ω 2 r 2 .
The surface is in contact with the atmosphere, so the pressure at every point on the surface is equal to the atmospheric pressure. Therefore, P A = P D = P a t m . We can start at A, or D and then find the pressure at D. Either way, we should get the same value for P C .
If we go from A → B → C , then P C = P a t m + ρ g a + 2 ρ ω 2 r 2
If we go from D → C , then P C = P a t m + ρ g b
From these two equations, we get:
ρ g a + 2 ρ ω 2 r 2 = ρ g b
b − a = 2 g ω 2 r 2
We see that the cross section of the surface of water is a parabola, since the height of the water is quadratic in r . The surface of water is actually obtained by revolving this shape about the axis, which is a paraboloid.