Square knocked down
The number in each small, blue square is the area of the square.
What is the area of the large, red square?
Source: Catriona Shearer
The answer is 135.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
8 solutions
This is a message to Ronald Chen : The puzzle is badly stated because as it is not stated that the 27 and 12 squares, on the right side, must be aligned on their right side, there are many possible answers (just build similar triangles with the 3, 12 and 27 squares and then adjust the length between the 12 square on the left side and the 27 square on the right right, so that the red square is connected as shown).
Log in to reply
You can tag a person by including "at" symbol next to the specified username.
Exactly, insufficient specification.
I solved it the exact same way you did it, Chew. Great solution.
Since g cd ( 3 , 1 2 , 2 7 ) = 3 , the area ratio 3 : 1 2 : 2 7 is equivalent to 1 : 4 : 9 , which transforms the given diagram to the following:
Figure 1.
Diagrams with primitive areas
Since the segments of the square are linear and perpendicular, the purple squares can be produced as shown:
Figure 2.
Lines extended to form purple squares; notice that the largest blue square extends outward and also shares the common vertex with the red square. Thereby, geometry easily shows that the lengths of the largest inner triangles are 3 and 6. Computation verifies that there is no other possible setup with different solutions, which follows similarity of triangles.
where the stack of 3 small squares can be shifted to position two smallest squares inside the largest rectangle. In this case, the result follows the area of the tangential square in the largest square of side length 6 + 3 = 9 :
Figure 3.
Resulting diagram from Figure 1
where the area of the inner square is 4 5 . Thus, Area of red square Area of smallest blue square = 4 5 1 = 1 3 5 3
which shows that the answer for this problem is 1 3 5 .
I really like this clean, efficient solution the best. Thank you.
Log in to reply
That is the most imaginative solution I came up with. :)
I admire the second step of extending lines to form squares! I guess, That it's the most intelligent way of solving!!🤔 Awesome solution @Michael Huang ! 😅
Relevant wiki: Pythagorean Theorem
 In the right hand side of the image, the area of blue square equal to 3, 12, and 27 and their corresponding side length equal to
3
,
2
3
,
a
n
d
3
3
respectively. Clearly, sum of the sides of the blue square equal to
6
3
which is equal to
black dotted vertical line
.
In the right hand side of the image, the area of blue square equal to 3, 12, and 27 and their corresponding side length equal to
3
,
2
3
,
a
n
d
3
3
respectively. Clearly, sum of the sides of the blue square equal to
6
3
which is equal to
black dotted vertical line
.
The blue square with side length of 1 3 placed in the middle of the blue square with side length 2 3 . Difference of sides equal to 2 3 - 1 3 equal to 1 3 . The length splitted as 2 ( 3 ) , 3 , and 2 ( 3 ) . Similarly, The blue square with side length of 2 3 placed top of blue square with side length 3 3 . Difference of sides equal to 3 3 - 2 3 equal to 1 3 . The length splitted as 3 , and 2 3 . The length of the black dotted horizontal line is 2 ( 2 ( 3 ) + 3 ) equal to 3 3 .
Using Pythagorean theorem, the length of red square is 1 3 5 , which is calculated using doted legs. Hence the area of the red square equal to 1 3 5 x 1 3 5 =135.
How do you know that the square with area 3 is horizontally placed exactly in the middle of the square with area 12?
Log in to reply
You use the fact that the triangles between the red square and blue squares are similar triangles. So between red square and blue square of area 12, the base is length sqrt(3). The lengths of the blue squares tell us the length scale factor from middle square to top square is 0.5. Consequently the triangle between red square and blue square of area 3 must have base length 0.5*sqrt(3).
[(√27 - √12)/√12] = (1/2) gives the cot(x) of the angle, where "x" is the inclination of the right side of the red square. Therefore, the red square area
= {(√3+√12+√27)^2}(1+cot(x)^2)
Answer=135
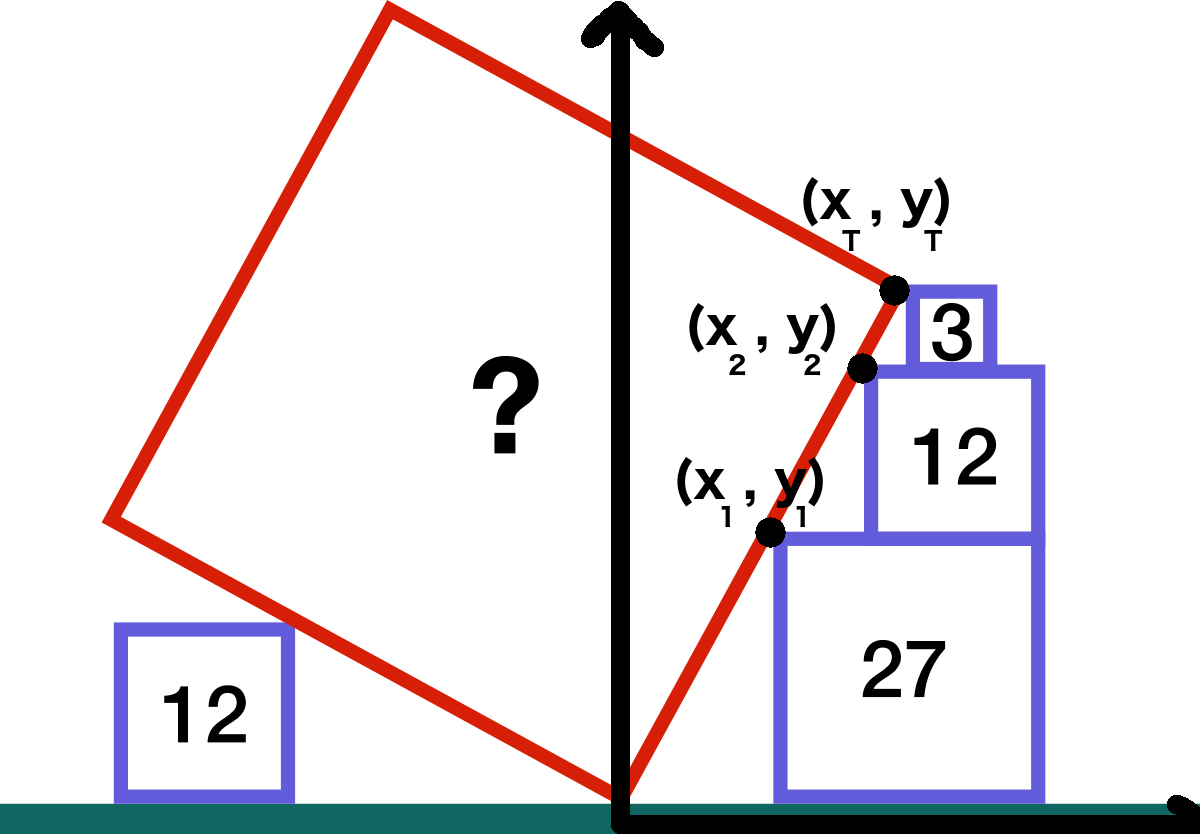 We can solve the problem algebraically
We can solve the problem algebraically
From the diagram we know that Y T = 3 3 + 2 3 + 3 = 6 3
To find X T we need to find the slope of the line
Slope = Δ X Δ Y = X 2 − X 1 Y 2 − Y 1 = 3 3 − 2 3 2 3 = 3 2 3 = 2
note: Δ X is the difference between the length of the side of the bottom square and the length of the side of the middle square and Δ Y is the length of the side of the middle square
Therefore X T = S l o p e Y T = 2 6 3 = 3 3
Area = S i d e 2
Area= S i d e 2 = Y T 2 + X T 2
Area= ( 6 3 ) 2 + ( 3 3 ) 2
Area= 1 3 5
how did you get x1 and x2?
Log in to reply
I didn’t get x1 and x2 individually. I got the “difference” between them. It is the length of side of the bottom square minus the length of the side of the middle square. Thanks for pointing this out I just added the note to the solution.
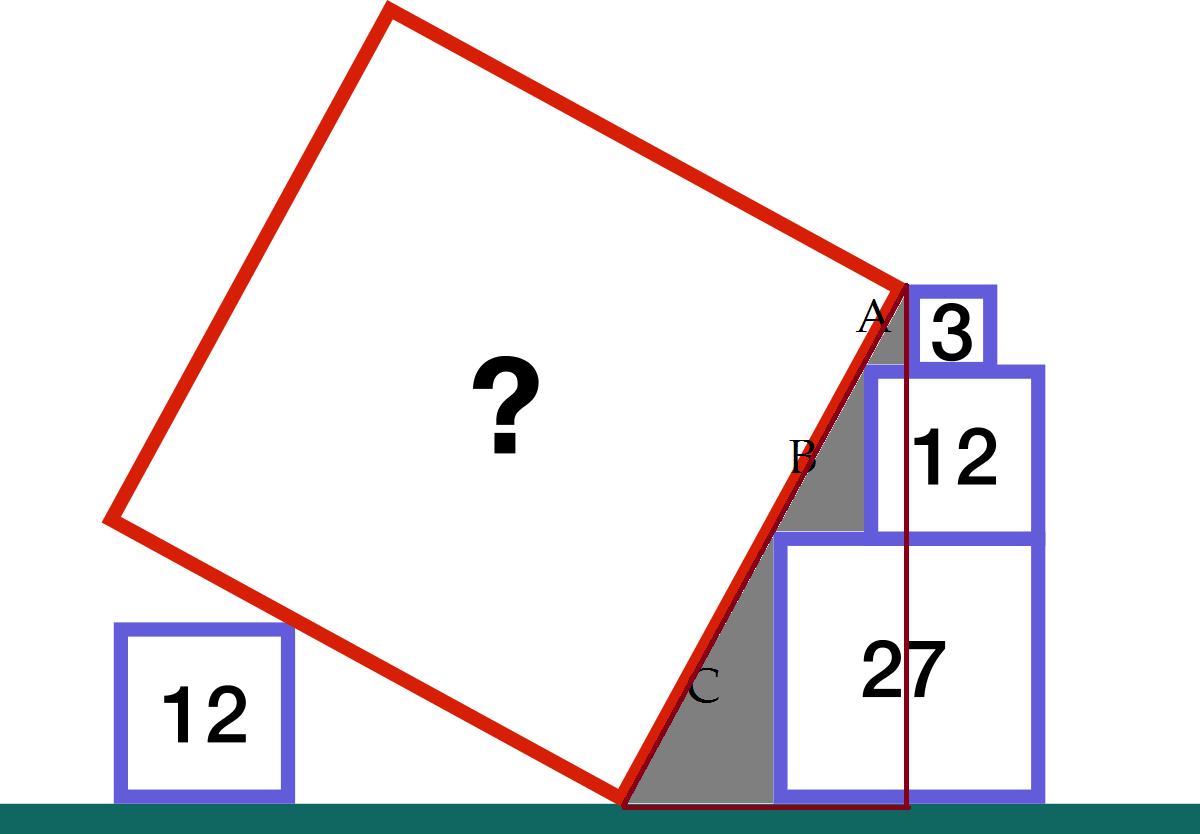
Similar triangles are the key! Let the triangles A,B,C be the three similar grey triangles marked in the picture. We know they are similar because the angle the red square's side makes with the blue squares' side is the same, and the angle opposite to the red square's side is always 90 degrees.
Notice that the heights of these triangles are 3 , 2 3 and 3 3 , respectively, implying that the three of them are such that C is three times as large as A, and B is twice as large as A. Moving on, notice that the lower-right vertex of the 12 square coincides with the upper-right vertex of the 27 square, implying that the base of triangle B is 2 7 − 1 2 = 3 , which, in turn, implies the base of triangle A is 2 3 and the base of triangle C is 3 2 3 .
Let's now turn our attention to the triangle marked in red. Notice that its height equals the sum of the side lengths of the 3, 12 and 27 squares, totaling 6 3 , and its base is the sum of the side lengths of A,B, and C, totaling 3 3 . We then apply pythagoras:
( 6 3 ) 2 + ( 3 3 ) 2 = l 2 = 1 3 5 where l is the side length of the red square, implying its area is 135.
Woops, forgot the picture:
Consider:
 Assume that the 12 and 27 squares are right aligned. Tan (phi) = a / b = sqrt(12)/[sqrt(27)-sqrt(12)] = 2
Assume that the 12 and 27 squares are right aligned. Tan (phi) = a / b = sqrt(12)/[sqrt(27)-sqrt(12)] = 2
Now consider:
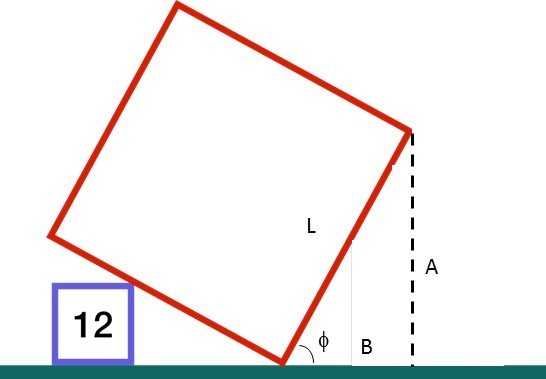 Area = L^2 = A^2 + B^2. From the above, A/B = 2, so Area = 5/4 A^2
Area = L^2 = A^2 + B^2. From the above, A/B = 2, so Area = 5/4 A^2
A = sqrt(3) + sqrt(12) + sqrt(27)
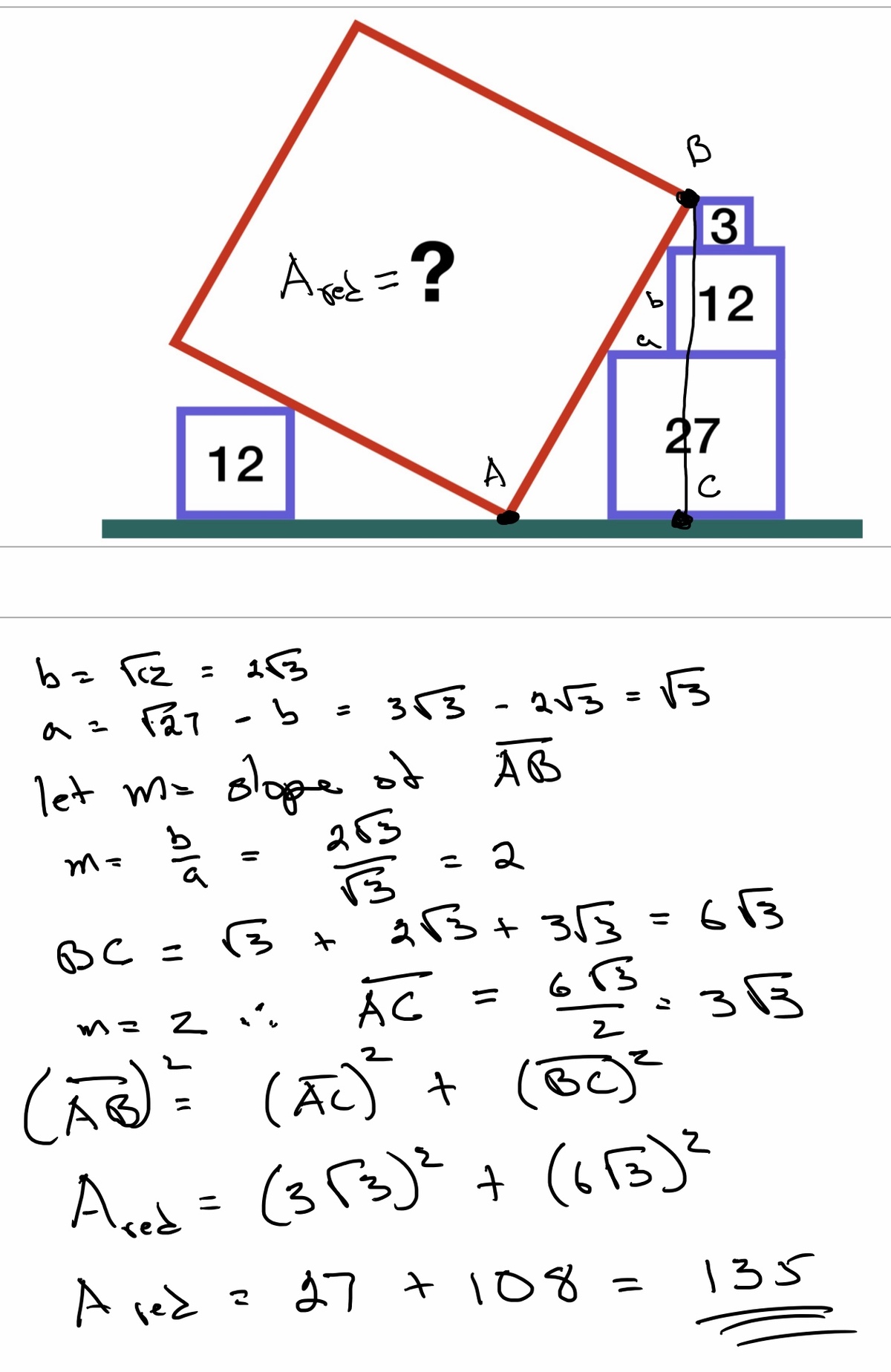
We note that the three grey right triangles in the figure are similar. The side lengths of the three squares on the right are 3 , 1 2 = 2 3 , and 2 7 = 3 3 . Therefore, the ratio of side lengths of the three similar grey right triangles is 1 : 2 : 3 .
Let the base length of the middle grey right triangle be a and its hypotenuse be b . Assuming the right edges of the 2 3 -square and 3 3 -square are aligned, then a = 3 3 − 2 3 = 3 . By Pythagorean theorem, we have b 2 = a 2 + ( 2 3 ) 2 = 3 + 1 2 = 1 5 ⟹ b = 1 5 .
Let the side length of the large square be s . Since the ratio of side lengths of the three similar grey right triangles is 1 : 2 : 3 , s = 2 1 + 2 + 3 b = 3 b = 3 1 5 and the area of the large square A = s 2 = ( 3 1 5 ) 2 = 1 3 5 .