Square Plus One

Number Theory Level 3

Consider the formula $x^2+1=p$ , where $p$ is a prime number and $x$ is a positive integer.

How many variables for $x$ less than or equal to 50 are there?

The answer is 12.

1 solution

Sarah Preston
Nov 14, 2016

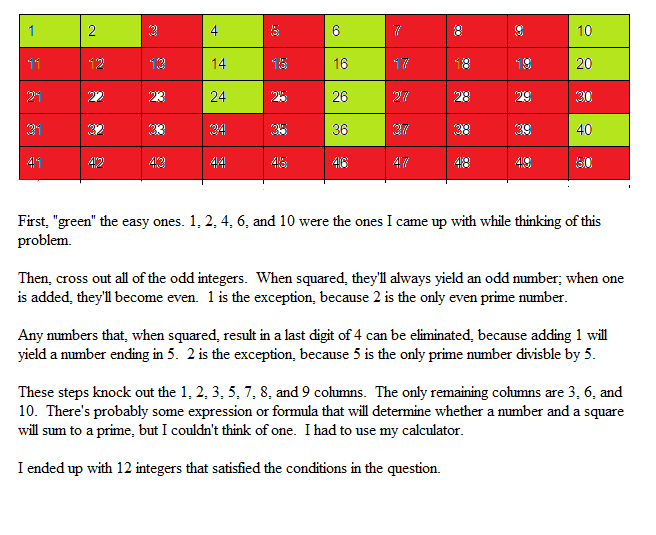
Nice observations that you made exploring this problem.

I don't think there is a general expression that will tell you easily if $x^2 + 1$ is a prime. We know that for cases, it cannot be a prime, like when $x$ is odd and larger than 1, or when $x = 5k+2 , 5k+ 3$ (with $k \geq1$ ).

Keep on investigating!

Calvin Lin Staff - 4 years, 7 months ago

I'm curious into investigating the $10k+6$ numbers, noticing that all of them currently listed yielded a prime. Is this always true or if not, what is the first number that fails?

P.S. Its obviously not true since primes aren't always within 10 of each other, but finding the first to fail of this would be interesting.

Seth Christman - 4 years, 7 months ago

Since we're looking at $(10k+6)^2 + 1$ which is a polynomial form, and we know that there isn't a polynomial that always produces prime numbers, hence the answer is not true.

There is a connection to gaussian integers , because you're asking when is $1 + ix, 1 - ix$ a Gaussian prime.

Note: As mentioned in the report, 46 is the first counter example.

Calvin Lin Staff - 4 years, 7 months ago

Square Plus One

The answer is 12.

1 solution

0 pending reports