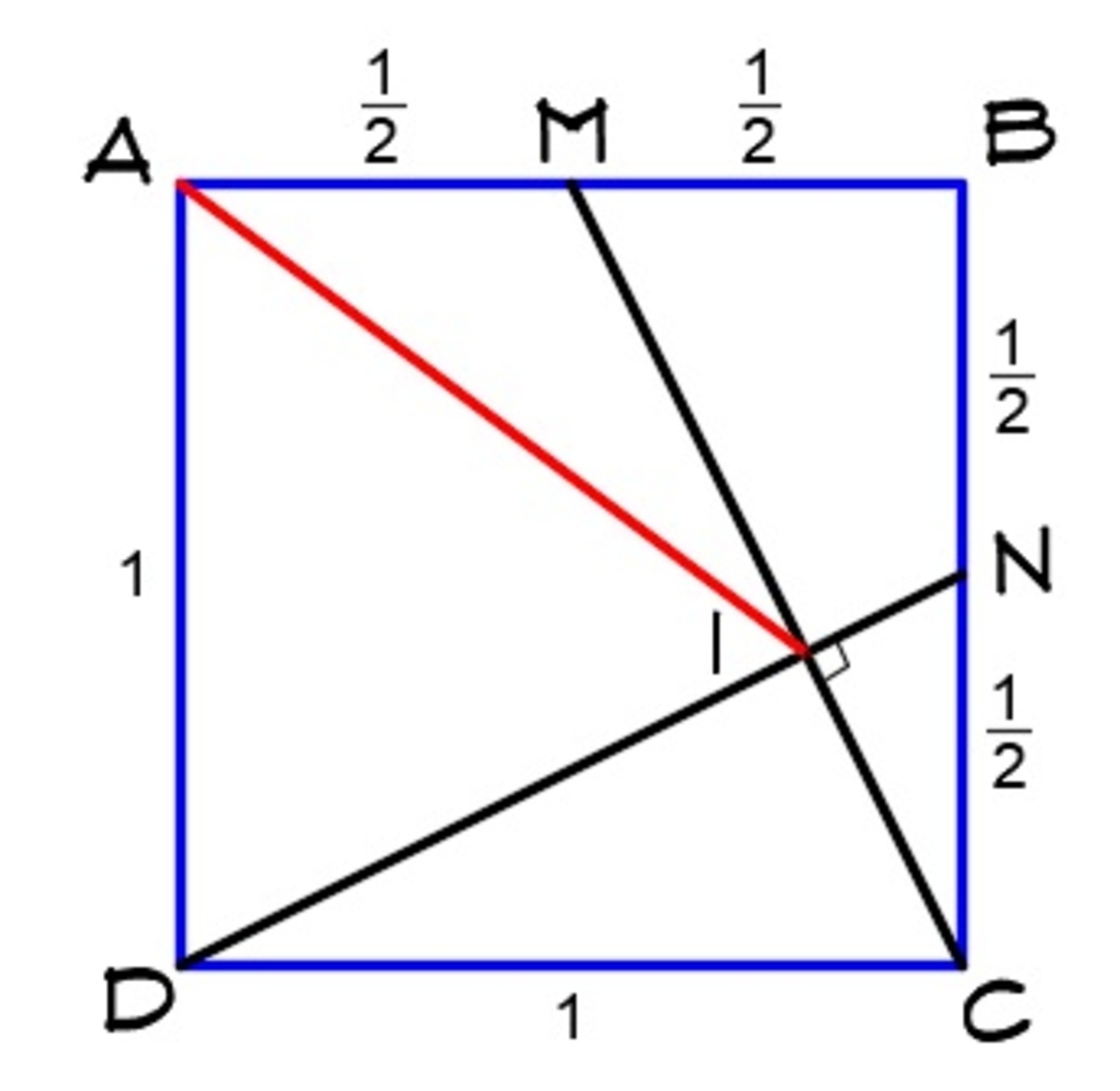
Squares and midpoints

We can easily prove that $\triangle BMC \cong \triangle CND (SAS)$ and therefore $CM\perp DN$ and $\angle MID=90^{\circ}$ .

Let $CM$ cut $AD$ at $E$ . We can also easily prove that $\triangle BMC \cong \triangle AME (ASA)$ and therefore $A$ is the midpoint of $DE$ . On the other hand, $\triangle DIE$ is a right triangle at $I$ and its median, $IA$ , starts from the right angle. We can conclude that $AI=\frac{1}{2}DE=AD=\boxed{1}$

The “slope” of $MC$ is -2 while the “slope” of $ND$ is $\frac{1}{2},$ so $MC \perp ND.$ This means quadrilateral $AMID$ is cyclic, since both $\angle MAD$ and $\angle MID$ are both right. Using congruent triangles $\triangle AMD \cong \triangle BMC$ and properties of cyclic quadrilaterals, we have

$\begin{aligned} \angle ADI &= 180^{\circ} - \angle AMI \\ &= \angle BMI \\ &= \angle AMD \\ &= \angle AID. \end{aligned}$

Thus, $\triangle AID$ is isosceles, giving us $AI = AD = \boxed{1}.$

By pythagorean theorem, $MC=\sqrt{1^2+\left(\dfrac{1}{2}\right)^2}=\sqrt{\dfrac{5}{4}}=\dfrac{1}{2}\sqrt{5}$ .

Since $\triangle NIC \sim \triangle MBC$ , we have

$\dfrac{IC}{NC}=\dfrac{BC}{MC}$ $\implies$ $\dfrac{IC}{\frac{1}{2}}=\dfrac{1}{\frac{1}{2}\sqrt{5}}$ $\implies$ $IC=\dfrac{1}{\sqrt{5}}=\dfrac{\sqrt{5}}{5}$

Then: $MI=MC-IC=\frac{1}{2}\sqrt{5}-\frac{1}{5}\sqrt{5}=\frac{3}{10}\sqrt{5}$

And: $\tan \angle BMC=\dfrac{BC}{BM}=\dfrac{1}{\frac{1}{2}}=2$ $\implies$ $\angle BMC = \tan^{-1}2$

It follows that: $\angle AMC = 180 - \angle BMC = 180 - \tan^{-1}$

Apply cosine rule on $\triangle AMI$ , we have

$(AI)^2=(AM)^2+(MI)^2-2(AM)(MI)\cos \angle AMC=\left(\dfrac{1}{2}\right)^2+\left(\dfrac{3}{10}\sqrt{5}\right)^2-2\left(\dfrac{1}{2}\right)\left(\dfrac{3}{10}\sqrt{5}\right)\left[\cos \left(180-\tan^{-1} 2 \right)\right]=1$

Finally, $AI=\sqrt{(AI)^2}=\sqrt{1}=\boxed{1}$

Use coordinate geometry with D(0,0). Equation of line DN is y=0.5x, MC: y=-2x + 2. I is intersection point of line DN and MC. So we can find its coordinate I(4/5,2/5). So by distance formula we can find AI = 1