Squares and Triangles
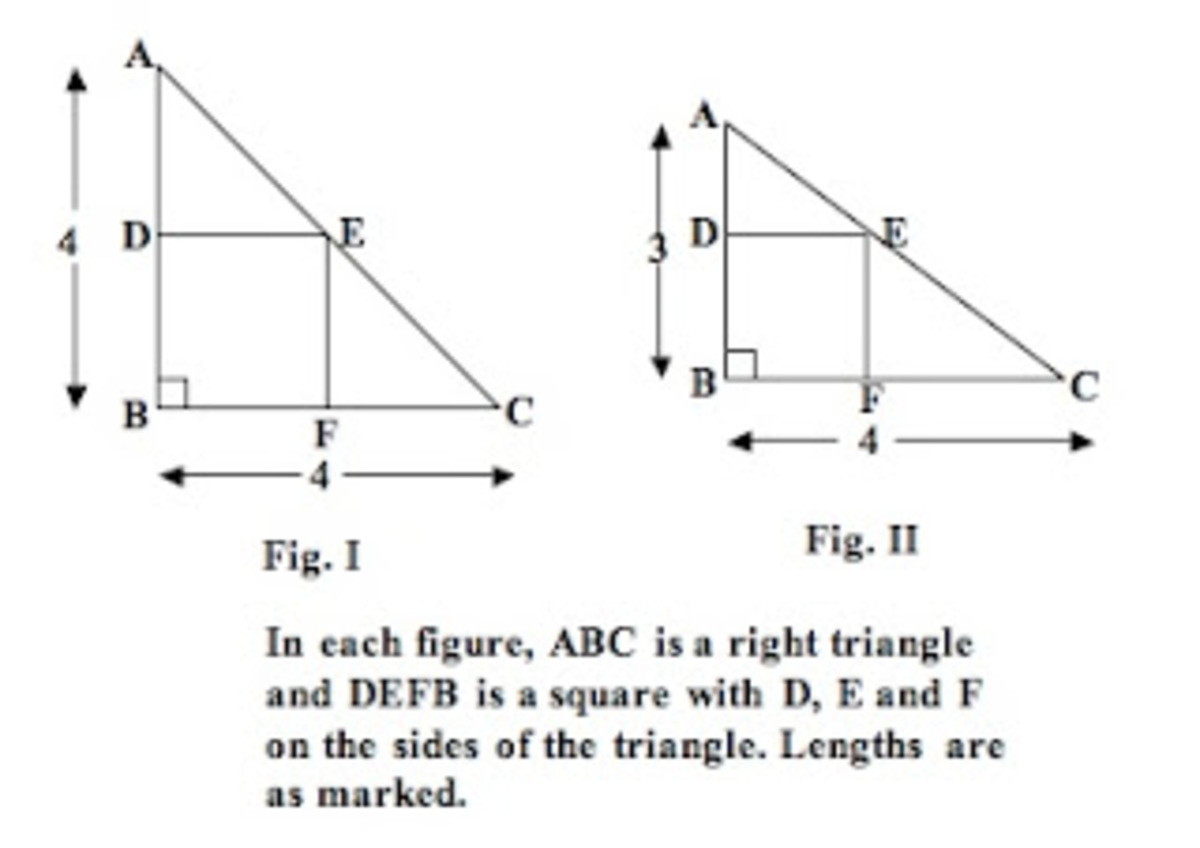
The length of the square in figure II is b a , where a and b are relatively prime. Find a + b .
The answer is 19.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Let the length of the square be x . A C = A B 2 + B C 2 = 3 2 + 4 2 = 5 . Employing similar triangles,
A E = 4 5 x while C E = 3 5 x , therefore:
4 5 x + 3 5 x = 5
4 x + 3 x = 1
3 x + 4 x = 3 × 4
( 3 + 4 ) x = 1 2
7 x = 1 2
x = 7 1 2
Therefore, a + b = 1 2 + 7 = 1 9
Δ ADE ~ Δ ABC
Therefore, given x is square length, we have 3 − x x = 3 4
and, finally, x=12/7
If we were to have catheti a and b, respectively, x would be given by formula
x = a + b a b