Squares in a semicircle

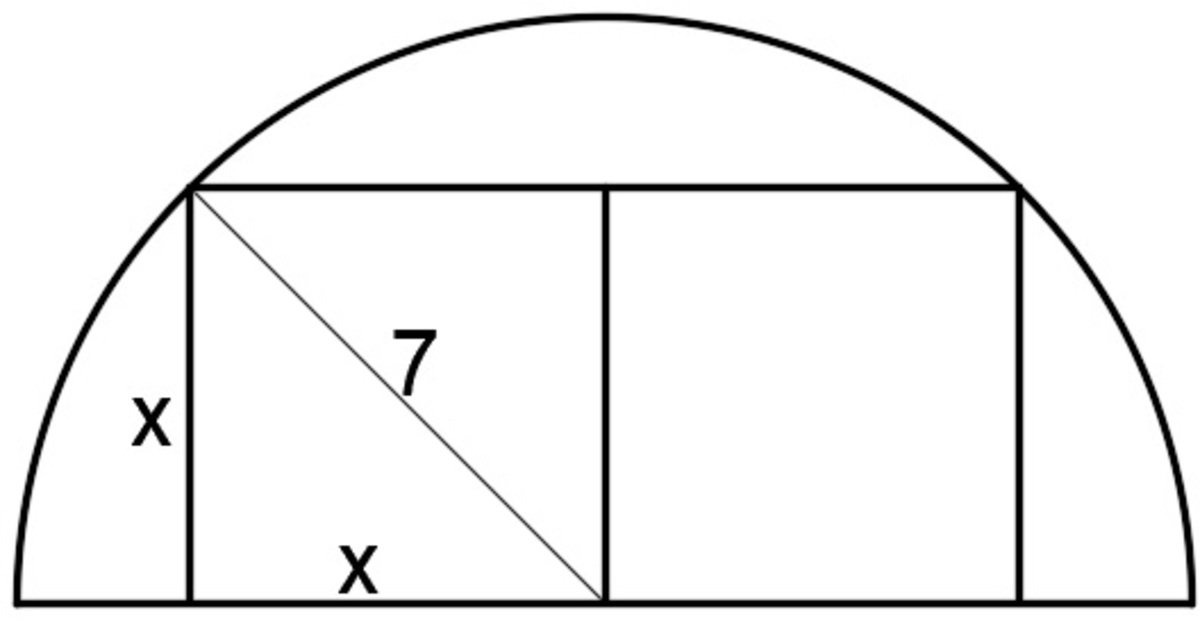
The squares have side lengths between $0$ and $7.$ Call the sides $7\sin \theta$ and $7 \sin \gamma,$ for $\theta,\gamma \in [0, \pi/2].$ Then the left square has height $7 \sin \theta$ and so point $A$ is $(-7 \cos\theta, 7 \sin \theta).$ Similarly point $B$ is $(7 \cos\gamma, 7 \sin\gamma).$ The difference between the $x$ -coordinates is the sum of the lengths of the two squares, so $7\cos \theta + 7\cos\gamma = 7\sin \theta + 7 \sin \gamma.$

Using addition formulas, we get $14\cos\left( \frac{\theta + \gamma}2 \right) \cos\left( \frac{\theta - \gamma}2 \right) = 14 \sin \left( \frac{\theta + \gamma}2 \right) \cos\left( \frac{\theta - \gamma}2 \right),$ so $\cos\left( \frac{\theta + \gamma}2 \right) = \sin\left( \frac{\theta + \gamma}2 \right),$ which means that $\theta + \gamma = \pi/2.$ That is, $\sin\theta = \cos\gamma$ and $\cos\theta = \sin\gamma$ (which makes it clear that the original equation is satisfied).

The sum of the areas of the squares is $49 \sin^2 \theta + 49 \sin^2 \gamma = 49 \sin^2 \theta + 49 \cos^2 \theta = \fbox{49}.$

We can redraw the given two squares into two congruent squares as shown. So the area of the two squares is $2x^2$ .

By Pythagorean Theorem,

$7^2=x^2+x^2$

$49=2x^2$

Answer: $\color{#69047E}\large{\boxed{49}}$