Star Moment
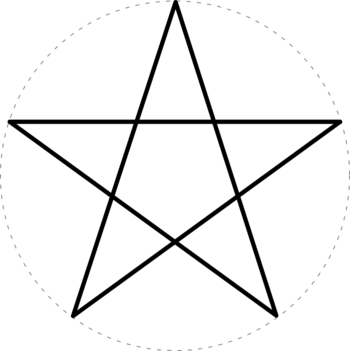
Consider a regular star inscribed in a unit circle, as shown in the figure above. The star wireframe has a uniform linear mass density, and the total mass of the star is M .
If the moment of inertia of the star about the axis, passing through its center and perpendicular to its plane, can be expressed as α M , determine the value of α to 3 decimal places.
The answer is 0.397.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Unit radii circle bro
How u got the angle bro??
Log in to reply
Since it is a regular pentagon, the angle is 5 3 6 0 ∘ = 7 2 ∘ .
Thnxx bro I didnt noticed ...that...🐱
How hypotenuse of the triangle is 1?
Use the expression for a rod about the center, along with the parallel axis theorem. From the figure, notice that the length of each of the 5 lines is 2 cos ( 1 8 ∘ ) , and that the distance of each line from the origin is sin ( 1 8 ∘ ) .
I = 5 ( 5 M 1 2 ( 2 cos ( 1 8 ∘ ) ) 2 + 5 M ( sin ( 1 8 ∘ ) ) 2 ) ≈ 0 . 3 9 7
Nice problem.
We can consider the star to be made of five rods, each with mass 5 M . Each rod will have the same moment of inertia about the center of the star, since the distance of each rod about the center is the same.
We will first find the length of one rod. Consider the right triangle formed by joining the center of the circle, the midpoint of a rod, and an endpoint of the rod.
The hypotenuse of the triangle is 1 , and the other sides have the length sin 7 2 ∘ and cos 7 2 ∘ . The length of the rod is ℓ = 2 sin 7 2 ∘ . Thus, the moment of inertia of each rod about its center is 1 2 m ℓ 2 = 5 M 1 2 ( 2 sin 7 2 ∘ ) 2 .
We want to find the moment of inertia of the rod about an axis which is at a distance d = cos 7 2 ∘ away from the center of the rod. Using the parallel axis theorem, the moment of inertia of the rod about the center of the circle I ′ is given by
I ′ = I + m d 2 = I + 5 M ( cos 7 2 ∘ ) 2 = 5 M 1 2 ( 2 sin 7 2 ∘ ) 2 + 5 M ( cos 7 2 ∘ ) 2
The moment of inertia of the complete star is
5 I ′ = M 1 2 ( 2 sin 7 2 ∘ ) 2 + M ( cos 7 2 ∘ ) 2 = M ( 3 sin 2 7 2 ∘ + cos 2 7 2 ∘ ) ≈ 0 . 3 9 7 M