Star - Part 3

Geometry Level 2

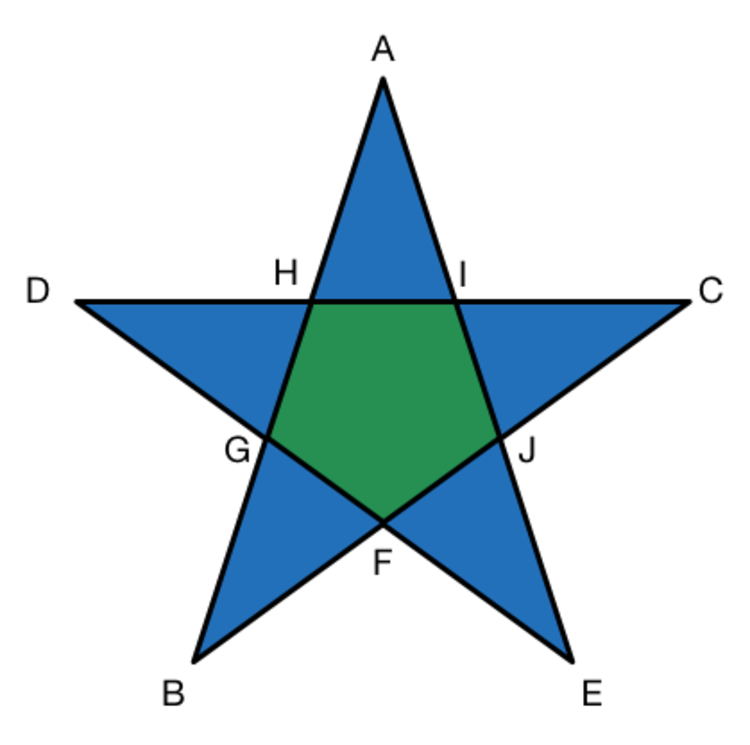The above image shows a 5-pointed star ABCDE, and a regular pentagon FGHIJ.

If the star has a (outside) perimeter of 50, and the pentagon has a perimeter of 30, what is the perimeter of AHI?

24 20 10 16

Chung Kevin by Chung Kevin , 45, Australia

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Phoebe Liu
Nov 8, 2015

The star has a perimeter of 50, and 10 "sides" that are equal in length, so each side is 50/10=5. So AH=5 and AI=5. The regular pentagon has a perimeter of 30, and 5 congruent sides, so each side is 30/5=6. So HI=6. The perimeter of AHI=length of AH+length of HI+length of AI= 5+5+6=16.

1 Helpful 0 Interesting 0 Brilliant 0 Confused

It seems to me that something is fishy.

If A H = 5 AH=5 and H I = 6 HI=6 , then H I > A H HI > AH . But for a five pointed star A H > H I AH > HI .

In fact, shouldn't A H H I \dfrac{AH}{HI} be the golden ratio, 1.618 1.618 ?

Geoff Pilling - 4 years ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...