Star Waltz
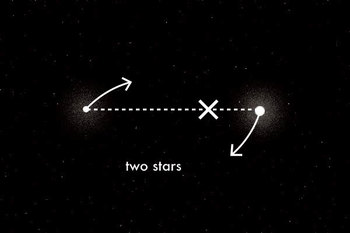 Two stars orbit their common center of mass as shown in the diagram below. The masses of the two stars are
3
M
and
M
. The distance between the stars is
d
. Determine the period of orbit for the star of mass
3
M
.
Two stars orbit their common center of mass as shown in the diagram below. The masses of the two stars are
3
M
and
M
. The distance between the stars is
d
. Determine the period of orbit for the star of mass
3
M
.
Note : This problem appeared on the 2009 F = ma exam.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
Discussions for this problem are now closed
You could have used d 2 G ( 3 M ) ( M ) = ( 3 M ) ( ω ) 2 4 d where ω is the angular frequency. It would have made the calculations easier and shorter.
Good point, although it is not obvious at first why F = M 0 ⋅ ω 2 ⋅ r 1 .
More simple write the Time period formula and replace the mass M with the reduced mass. Reduced mass = m1*m2/(m1+m2)
grt explanation!!
First of all, we need to find the centre of mass of the given system.
CENTER OF MASS
Assume the stars to be point objects. Let the mass M be at origin i.e. ( 0 , 0 ) . Since the distance between the stars is d , the star with mass 3 M will be at ( d , 0 ) . The center of mass ( x c , y c ) can now be computed as:
( x c , y c ) = ( M + 3 M M × 0 + 3 M × d , M + 3 M M × 0 + 3 M × 0 ) = ( 4 3 d , 0 ) .
TIME PERIOD
We know that for an object revolving around a point at a distance R with a velocity v , the time period can be computed using:
T = v 2 π R ...........................(1)
So now, we have to find v .
We know that in rotational motion, a = R v 2 . Also, a = m a s s F ⇒ R v 2 = m a s s F .
Substituting R = 4 d , F = d 2 G ( M ) ( 3 M ) , we get
4 d v 2 = d 2 3 M 3 G M 2 ⇒ v = 2 1 d G M .
Substituting this in equation (1), we get:
T = 2 1 d G M 2 π 4 d
or T = π G M d 3
Used the same approach previously but lost this time :P
Two stars in a binary system are bound by gravity and revolve around a common center of mass. In this case, we may be treated as if all its mass the system is concentrated at its center of mass, which is M ′ = M + 3 M = 4 M . Therefore, by using Newton's Law of gravity we obtain F m a a ω 2 d T 2 π T = R 2 G M ′ m = d 2 G ( 4 M ) m = d 2 4 G M = d 2 4 G M = d 3 4 G M = π G M d 3 For extra information, this problem can also be solved by using Kepler's 3 r d Law. # Q . E . D . #
What you have actually used is the reduced mass method.
Let,
F =gravitational force between the two stars (=G d 2 ( m 1 ) ( m 2 ) )
ω a & ω b =angular velocities of larger(3M) and smaller(M) stars respectively
r a & r b =distance of larger and smaller stars from the common centre of mass
Stars are in an orbit about their common center of mass. So we have
gravitational force(inwards) = centrifugal force(outwards)
Using this,
For larger star
G d 2 ( 3 M ) ( M ) =3M ω a 2 r a
=> ω a = d 2 r a G M -----(1)
For smaller star
G d 2 ( 3 M ) ( M ) =M ω b 2 r b
=> ω b = d 2 r b 3 G M
Now,
Since the common centre of mass does not change its position, we have that, time taken to complete one orbit for both stars is equal.
i.e, t a = t b
=>(2 π / ω a )=(2 π / ω b )
=> ω a = ω b
=> d 2 r a G M = d 2 r b 3 G M
=>3 r a = r b
Also we have r a + r b =d
=> r a = 4 d
Substituting above value of r a in eqn (1), we have
ω a = d 2 r a G M = d 3 4 G M
Hence,
t a =(2 π / ω a )= π G M d 3 ANS
Let,
r 1 = The distance of 3 M from the Center of Mass
r 2 = The distance of M from the Center of Mass
It is clear that,
r 1 + r 2 = d
3 M r 1 = M r 2
⇒ r 1 = 4 d and r 2 = 4 3 d
Also, writing the Force equation for 3 M ,
d 2 G ( M ) ( 3 M ) = r 1 ( 3 M ) v 1 2
Using the derived equations,
v 1 = 4 d G M
Thus,
w = r 1 v 1 = d 3 4 G M
We know,
T = w 2 π = π G M d 3