Stationary Distribution

Probability Level 2

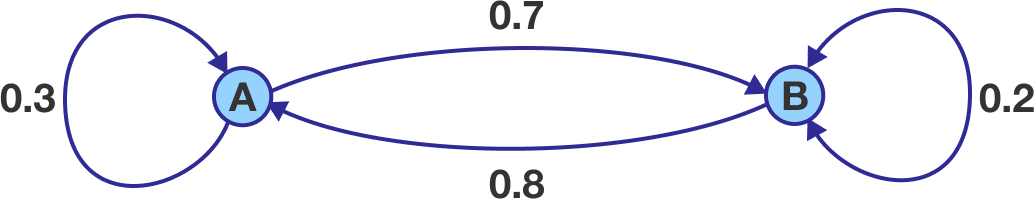
Find a stationary distribution for the 2-state Markov chain with stationary transition probabilities given by the following graph:

\left(\frac{1}{2}, \ \frac{1}{2}\right)

\left(\frac{8}{15}, \ \frac{7}{15}\right)

\left(\frac{3}{5}, \ \frac{2}{5}\right)

(1, \ 0)

2 solutions

John Storment
Apr 11, 2017

0.3 p + 0.8 (1 - p) = p
p = $\frac{7}{15}$
0.2 (1 - p) + 0.7 p = 1 - p
p = $\frac{8}{15}$

Solve for p in both equations

In this problem both the equation results are same ,i.e. p=8/15 How you calculate p=7/15 ??????? Please explain it.

TAMALENDU DAS - 3 years, 9 months ago

You are right about the solutions to the equations. The values needed in the answer are p and 1-p

Samir Betmouni - 3 years, 9 months ago

I looked at John's solution and realize that you just use the long run proportion property right???

xi chen - 3 years, 7 months ago

Although I chose the correct answer, I saw the incorrect message. Can you fix this problem? Thank you,

Mehmet Savasci - 3 years ago

Henry Maltby
Apr 28, 2016

The transition matrix is $\textbf{P} = \begin{pmatrix} 0.3 & 0.7 \\ 0.8 & 0.2 \end{pmatrix}$ . The matrix $\textbf{P}^T$ has eigenvector $(\tfrac{8}{7} \ 1)$ with eigenvalue 1. Therefore, a stationary distribution is $\boxed{(\tfrac{8}{15} \ \tfrac{7}{15}).}$ .

Fool me. It should be the TRANSPOSE of P.

Elva Lin - 3 years, 9 months ago

Forgot taking the transpose.

Sumit Lahiri - 7 months, 2 weeks ago

Why do I get a eigenvalues of 1 and -0.5???

Shuyu Lin - 3 months ago

It's correct. The eigenvectors you get from each will show you the answers. With eigenvalue -0.5, you get a negative probability, therefore you can eliminate that option. With eigenvalue 1, you get the shown answer.

Aras Senova - 1 month, 3 weeks ago

Stationary Distribution

2 solutions

1 pending report

Vote up reports you agree with