Stick's triangles
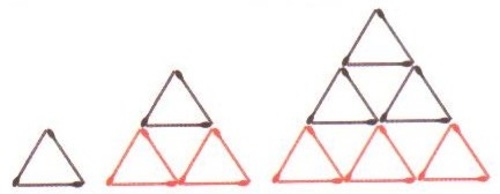 It was made a serie of stick's triangles following the sequence in the figure. One of these triangles was built with
sticks. How many sticks form the side of this triangle?
It was made a serie of stick's triangles following the sequence in the figure. One of these triangles was built with
sticks. How many sticks form the side of this triangle?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The number of triangles increases by the previous increase plus one unit, and becomes the triangular number . Since each triangle is built with three sticks, the formation pattern is given by:
S ( n ) = 2 3 . n . ( n + 1 ) .
Then, we have:
1 3 5 = 2 3 . n . ( n + 1 ) → n 2 + n − 9 0 = 0 → n = 9 .
Note : the other root of the polynomial is negative and, thus, discarded.