Strange region

There are two good ways to solve this problem. The first is to note that the region can be broken up into three parts, one of which is defined by $\{0 < x < 2 -y ~|~ 0 < y \leq 1 - \frac{1}{\sqrt{2}}\}$ , the second of which is $\{0 < x < \frac{1}{2y} ~|~ 1 - \frac{1}{\sqrt{2}} < y < 1 + \frac{1}{\sqrt{2}}\}$ , and the third of which is $\{0 < x < 2 -y ~|~ 1 + \frac{1}{\sqrt{2}} \leq y < 2\}$ . We then perform three double integrals $\displaystyle \iint \text{dA}$ with these limits to obtain an answer of $A = -\sqrt{2}+2+\frac{1}{2} \log \left(3+2 \sqrt{2}\right) \approx 1.467 \Rightarrow \left\lfloor100A\right\rfloor = \boxed{146}$ .

However, I'd like to introduce a different manner of calculating the area that could be more useful in another context where the region provided cannot be so easily broken up. The differential form $\text{dA}$ is expandable to $\text{dx} \wedge \text{dy}$ , the wedge product of differentials $\text{dx}$ and $\text{dy}$ . Define $u$ and $v$ such that $u = 2xy$ and $v = \frac{x+y}{2}$ . Then, solve for $x$ and $y$ to get $x = v \pm \sqrt{v^2 - u/2}$ and $y = v \mp \sqrt{v^2 - u/2}$ . For each solution $x$ and $y$ , the region defined by $0 < u < 1$ and $0 < v < 1$ is half of the problem region, as the two regions are symmetric over the line $y = x$ . Take the first solution $x$ and $y$ . We find that $\text{dx} = \frac{1}{4 \sqrt{v^2-u/2}} \text{du} + \left(1 + \frac{v}{\sqrt{v^2-u/2}}\right) \text{dv}$ and $\text{dy} = -\frac{1}{4 \sqrt{v^2-u/2}} \text{du} + \left(1 + \frac{v}{\sqrt{v^2-u/2}}\right) \text{dv}$ . Then, $\text{dx} \wedge \text{dy} = \left(\frac{1}{4 \sqrt{v^2-u/2}} \text{du} + \left(1 + \frac{v}{\sqrt{v^2-u/2}}\right) \text{dv}\right) \wedge \left(-\frac{1}{4 \sqrt{v^2-u/2}} \text{du} + \left(1 + \frac{v}{\sqrt{v^2-u/2}}\right) \text{dv}\right)$ . Note that the wedge product is distributive and anticommutative, and that $\text{du} \wedge \text{du} = \text{dv} \wedge \text{dv} = 0$ . So, $\text{dx} \wedge \text{dy} = \left(\frac{1}{4 \sqrt{v^2-u/2}}\right)\left(1 + \frac{v}{\sqrt{v^2-u/2}}\right) \text{du} \wedge \text{dv} + \left(1 + \frac{v}{\sqrt{v^2-u/2}}\right)\left(-\frac{1}{4 \sqrt{v^2-u/2}}\right) \text{dv} \wedge \text{du} = \left(\frac{1}{4 \sqrt{v^2-u/2}}\right)\left(1 + \frac{v}{\sqrt{v^2-u/2}}\right) \text{du} \wedge \text{dv} - \left(1 + \frac{v}{\sqrt{v^2-u/2}}\right)\left(-\frac{1}{4 \sqrt{v^2-u/2}}\right) \text{du} \wedge \text{dv} = \frac{1}{2 \sqrt{v^2-u/2}} \text{du} \wedge \text{dv}$ .

So, $\displaystyle \iint \text{dA} = \iint \text{dx} \wedge \text{dy} = \iint \frac{1}{2 \sqrt{v^2-u/2}} \text{du} \wedge \text{dv}$ .

We then perform the integral $\displaystyle \int _0^1\int _0^1\frac{1}{2\sqrt{v^2-u/2}}\text{du} \, \text{dv}$ and multiply by $2$ (because, remember, this is one of two symmetric regions) to get the same answer as before, $A = -\sqrt{2}+2+\frac{1}{2} \log \left(3+2 \sqrt{2}\right) \approx 1.467 \Rightarrow \left\lfloor100A\right\rfloor = \boxed{146}$ .

$A=2-\int_{\displaystyle 1-\frac{1}{\sqrt{2}}}^{1+\frac{1}{\sqrt{2}}} (2-x)-\frac{1}{2x} dx=1.467...$

So, $\displaystyle \lfloor 100A \rfloor =146$

Suggestion to Michael: For Calculus problems such as these, where the wording is very clear, it acts against the difficulty of your problem if you give the graph. This should be left for the solver to do, as that is part of the problem.

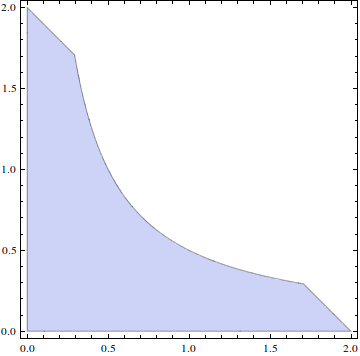
My Solution : So, given the diagram, we want to find the area of the first part, governed by the line, and the second part, governed by the curve, and the third part, re-governed by the line. For this, we need the two intersection points of the graphs of $xy = \tfrac{1}{2}$ and $x + y = 2$ .

We get that the $x$ -coordinates for the two intersection points are $1 - \dfrac {\sqrt{2}}{2}$ and $1 + \dfrac {\sqrt{2}}{2}$ . Thus, the area is

$A = \displaystyle\int_{0}^{1-\dfrac{\sqrt{2}}{2}} (2-x) \, \mathrm{d}x + \displaystyle\int_{1+\dfrac{\sqrt{2}}{2}}^{2} (2-x) \, \mathrm{d}x + \displaystyle\int_{1-\dfrac{\sqrt{2}}{2}}^{1+\dfrac{\sqrt{2}}{2}} \left( \dfrac {1}{2x} \right) \, \mathrm{d}x.$

Evaluating this is the easy part. Multiplying this by $100$ and taking the floor, we get $\boxed {146}$ .

The intersection points of the line and the hyperbolic region are given by, ((1-1/sqrt(2)) , (1+1/sqrt(2))) and ((1+1/sqrt(2)) , (1-1/sqrt(2)))

The given area A is simply divided into the following three parts:

1. Integral of (-x+2) from 0 to (1-1/sqrt(2))

2. Integral of (1/(2x)) from (1-1/sqrt(2)) to (1+1/sqrt(2))

3. Integral of (-x+2) from (1+1/sqrt(2)) to 2

Calculating the areas under the three regions and adding will give us the total area A bounded between the given inequalities...

We can find the area by taking the area between the curves $y = -x + 2$ and $y = \frac{1}{2x}$ and then subtracting that from $\frac{1}{2}(2)(2) = 2$ . Clearly the former function is on top. We need to also find their points of intersection.

$-x + 2 = \frac{1}{2x} \rightarrow 2x^2 - 4x + 1 = 0 \rightarrow 2(x - 1)^2 = 1$

\((x-1)^2 = \frac{1}{2}

\(x - 1 = \pm \frac{1}{\sqrt{2}}\)

$x = 1 \pm \frac{1}{\sqrt{2}}$

So now we know our limits of integration. Now, we can integrate.

$\int -x + 2 - \frac{1}{2x} dx$

$\large{[\frac{-x^2}{2} + 2x - \frac{1}{2} \ln x]_{1 - \frac{1}{\sqrt{2}}}^{1 + \frac{1}{\sqrt{2}}}}$

Using a calculator to find this value, we get a final answer of $146$ .