This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Nice solution. Didn't know it could be solved like this.
Same reasoning!
Wayyyy to complicate!! But congrats on finding a different way to reach the solution!!
Use the relation ( a + b ) 3 = a 3 + b 3 + 3 a b ( a + b ) to get y 3 = ( 3 3 + 3 3 1 ) 3 = 3 + 3 1 + 3 y = 3 1 0 + 3 y ⟹ 3 y 3 − 9 y = 1 0 .
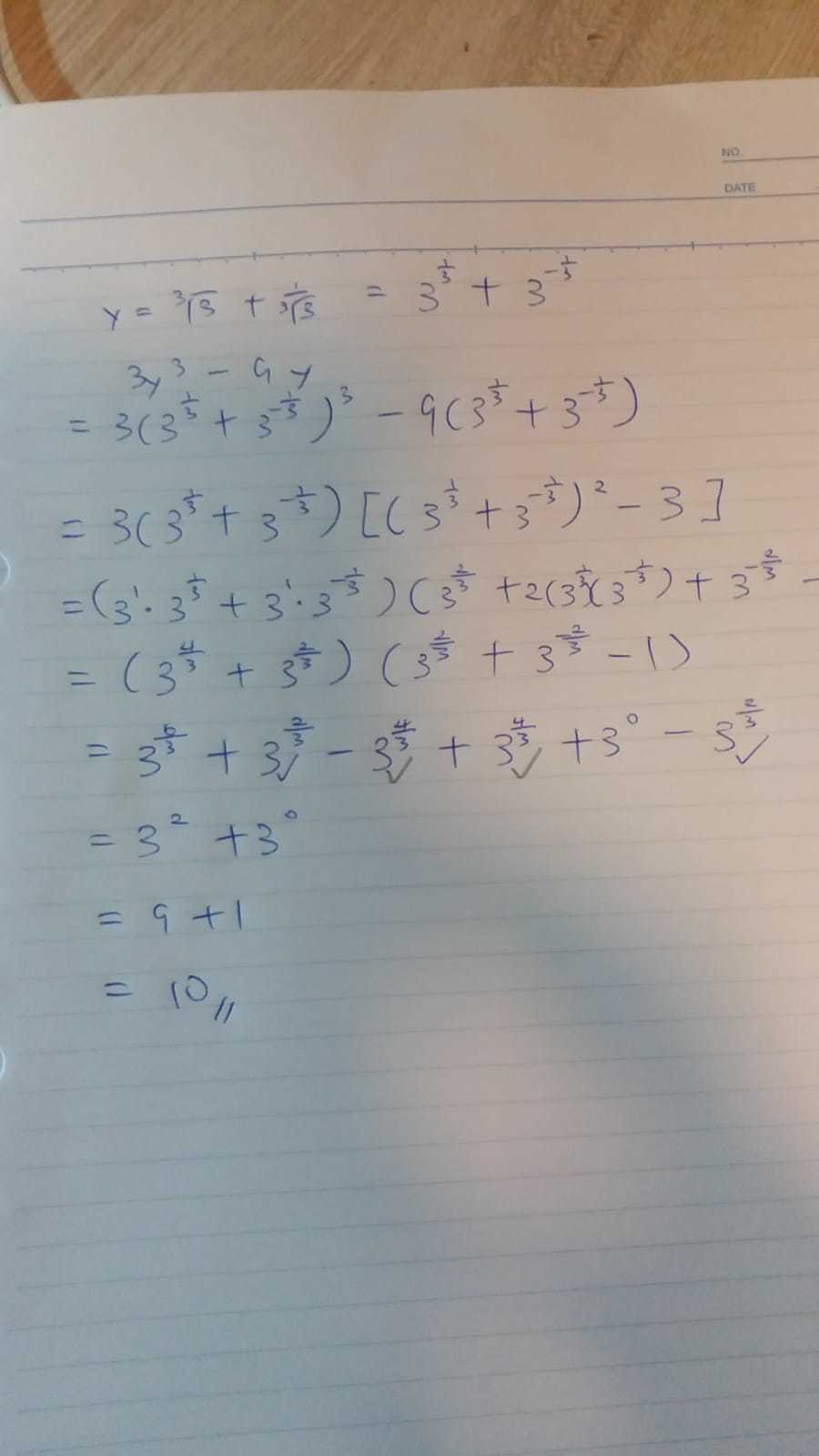
y y 3 y 3 3 y 3 = 3 3 + 3 3 1 = 3 + 3 3 3 + 3 3 3 + 3 1 = 3 + 3 y + 3 1 = 9 + 9 y + 1 Cubing both sides Multiplying both sides by 3 Rearranging
⟹ 3 y 3 − 9 y = 1 0