Successive Successful Squares
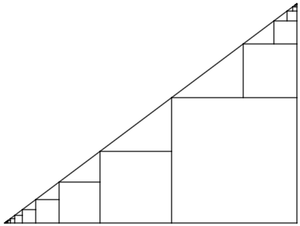
In the right triangle of sides , and , the largest square is positioned by the right angle. The squares are then drawn successively and infinitely on both sides of the squares as shown above.
If the area sum of all squares can be expressed as , where and are positive integers and , input as your answer.
The answer is 16237.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let the side length of the largest square be a . Consider the 3-4-5 triangle up top of the largest square. We note that
a 3 − a = 4 3 ⟹ 1 2 − 4 a = 3 a ⟹ a = 7 1 2
Let a 0 = a and the subsequent side lengths of the squares on top of the largest square be a n . Then we note that, for n ≥ 0 , a n a n + 1 = 4 7 1 2 = 7 3 . The area of squares of the largest square and those on top is:
n = 0 ∑ ∞ a n 2 = n = 0 ∑ ∞ ( 7 3 ) 2 a 0 2 = 1 − 4 9 9 1 ⋅ 4 9 1 4 4 = 4 0 1 4 4
Similarly, let b 0 = a and the subsequent side lengths of the squares on the left of the largest square be b n . Then we note that, for n ≥ 0 , b n b n + 1 = 3 7 1 2 = 7 4 . The area of squares of the largest square and those on the right is:
n = 0 ∑ ∞ b n 2 = n = 0 ∑ ∞ ( 7 4 ) 2 b 0 2 = 1 − 4 9 1 6 1 ⋅ 4 9 1 4 4 = 3 3 1 4 4
Then the area of all squares within the triangle:
A = n = 0 ∑ ∞ a n 2 + n = 0 ∑ ∞ b n 2 − a 2 = 4 0 1 4 4 + 3 3 1 4 4 − 4 9 1 4 4 = 2 6 9 5 1 3 5 4 2
Therefore the required answer 1 3 5 4 2 + 2 6 9 5 = 1 6 2 3 7 .