Sum of areas
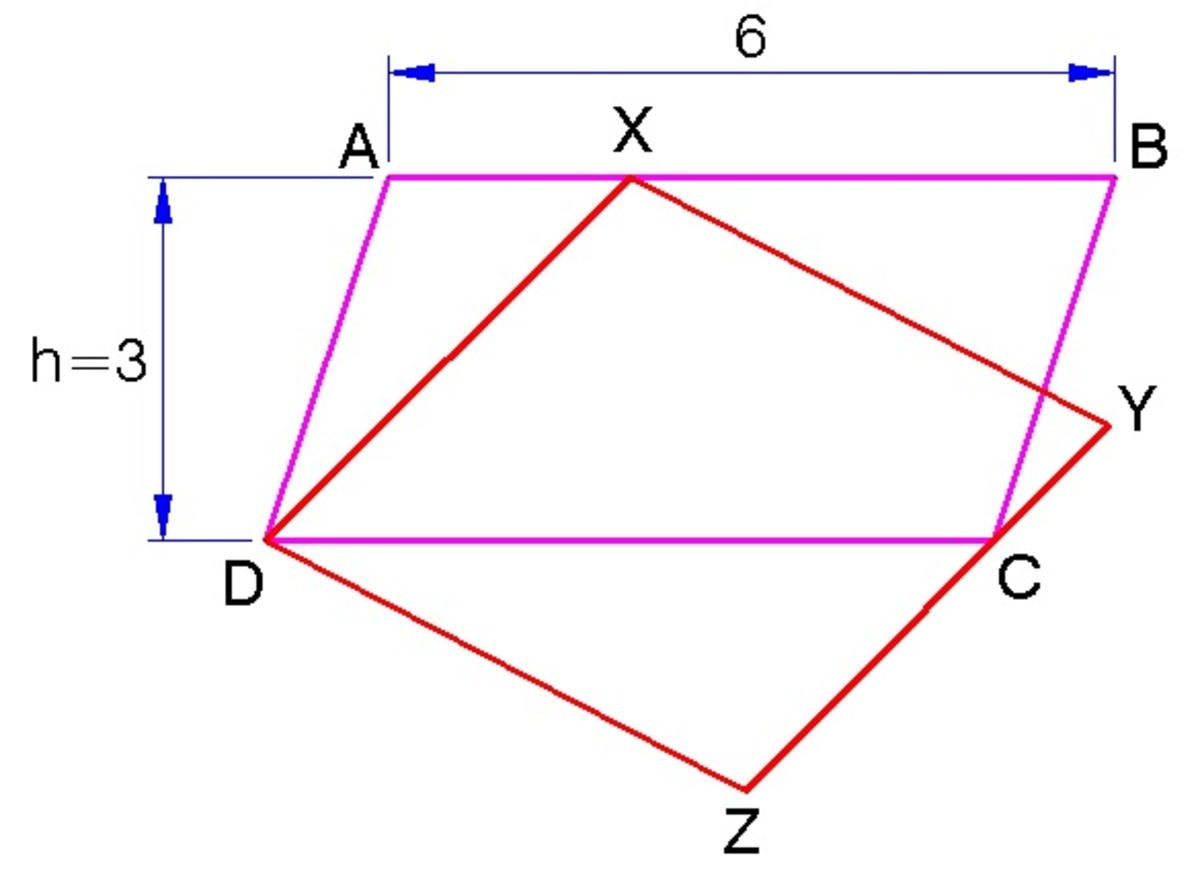 A
B
C
D
and
X
Y
Z
D
are both parallelograms. The height and base of parallelogram
A
B
C
D
are
3
and
6
, respectively. Point
X
lies on
A
B
and point
C
lies on
Y
Z
. What is the sum of the areas of triangle
X
Y
C
and triangle
D
C
Z
?
A
B
C
D
and
X
Y
Z
D
are both parallelograms. The height and base of parallelogram
A
B
C
D
are
3
and
6
, respectively. Point
X
lies on
A
B
and point
C
lies on
Y
Z
. What is the sum of the areas of triangle
X
Y
C
and triangle
D
C
Z
?
The answer is 9.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Original parallelogram: A r e a ( A B C D ) = 3 × 6 = 1 8
Triangle within that parallelogram with the same base and height: A r e a ( D X C ) = 2 1 × A r e a ( A B C D ) = 9
Same triangle sharing base and height with another parallelogram: A r e a ( D X C ) = 2 1 × A r e a ( D X Y Z ) ⟹ A r e a ( D X Y Z ) = 2 × 9 = 1 8
The area we are looking for as the area of the parallelogram minus the area of the triangle:
A r e a ( X Y C ) + A r e a ( D C Z ) = A r e a ( D X Y Z ) − A r e a ( D X C ) = 9
Draw X C . Since triangle X C D and parallelogram A B C D share the same base ( D C ) and a common altitude (from X to D C ), the area of triangle X C D is equal to one-half the area of parallelogram A B C D .
Similarly, triangle X C D and parallelogram X Y Z D share the same base X D and the same altitude to that base (from C to X D ) ; thus the area of triangle X C D is equal to one-half the area of parallelogram X Y Z D . Therefore, the area of the two parallelograms are equal.
A r e a ( A B C D ) = A r e a ( X Y Z D ) = 3 ( 6 ) = 1 8
Therefore,
A r e a ( X Y C ) + A r e a ( D C Z ) = 2 1 A r e a ( X Y Z D ) = 2 1 ( 1 8 ) = 9 answer