Sum of red = Sum of blue?
The figure below is made on the regular grid.
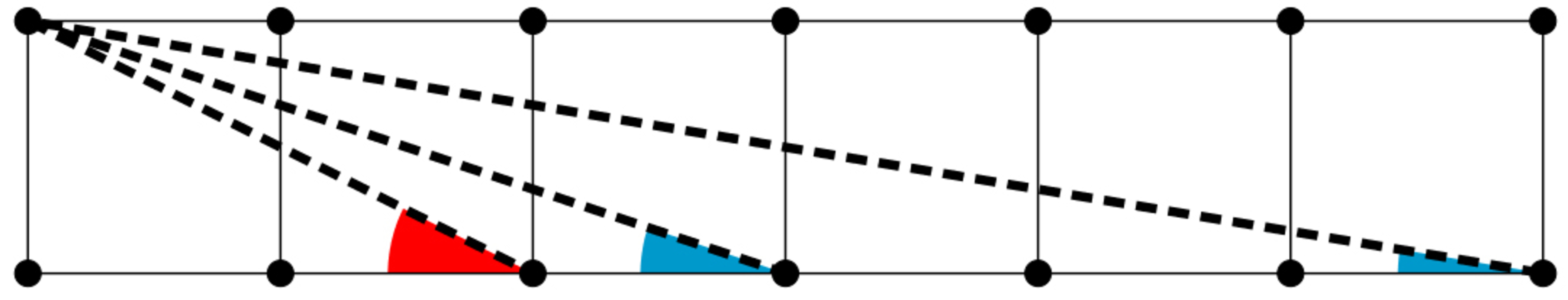
Which is bigger, the red angle, or the sum of the blue angles?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
I suppose that last calculation should be tan ( θ b 1 + θ b 2 ) = 1 8 1 7 2 1 = 1 7 9 .
The final result is still the same as 2 1 < 1 7 9 . :)
Assuming each grid side length equals unity, we have:
θ r e d = a r c t a n ( 2 1 ) , θ b l u e 1 = a r c t a n ( 3 1 ) , θ b l u e 2 = a r c t a n ( 6 1 ) .
Knowing that the tangent function is an increasing function, let's now calculate:
t a n ( θ r e d ) = 2 1 .
t a n ( θ b l u e 1 + θ b l u e 2 ) = 1 − t a n ( θ b l u e 1 ) t a n ( θ b l u e 2 ) t a n ( θ b l u e 1 ) + t a n ( θ b l u e 2 ) = 1 − ( 3 1 ) ( 6 1 ) 3 1 + 6 1 = 1 7 9 .
Since 2 1 < 1 7 9 , the blue angle sum is greater.