Sum of the terms
Number Theory
Level
2
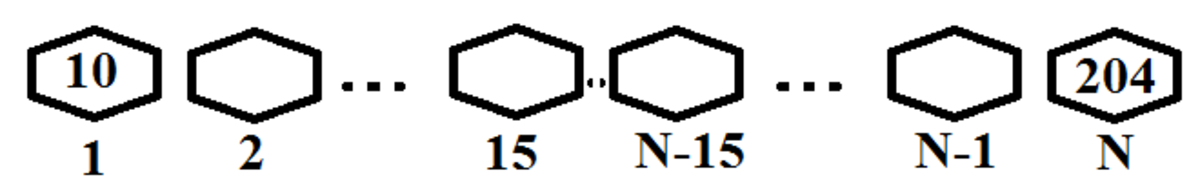A sequence of numbers that are in arithmetic progression, starts with 10 and ends with 204. What is the sum of the 15th term from the beginning of the sequence and 15th term from the end of the sequence?
The answer is 214.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
Relevant wiki: Arithmetic and Geometric Progressions Problem Solving
The quick way to solve is, if we know the property
"The sum of the mirror image term of an AP is always equal to the sum of the first and last term"
(i.e) 10+204=214.
(or)
1st term=10, 2nd term=10+d,....,15th term=10+14d
Nth term=204,(N-1)th term=204-d,....,(N-14)th term=204-14d
Therefore, (10+14d)+(204-14d)=214