Summing areas of triangles
In the figure, the corners of each square are exactly the midpoint of the line. The squares continue until they are infinitely small.
What is the sum of
a
and
b
where
b
a
is the
exact
difference in area between the
shaded region
and the
non-shaded
region?
a
and
b
are relatively prime.
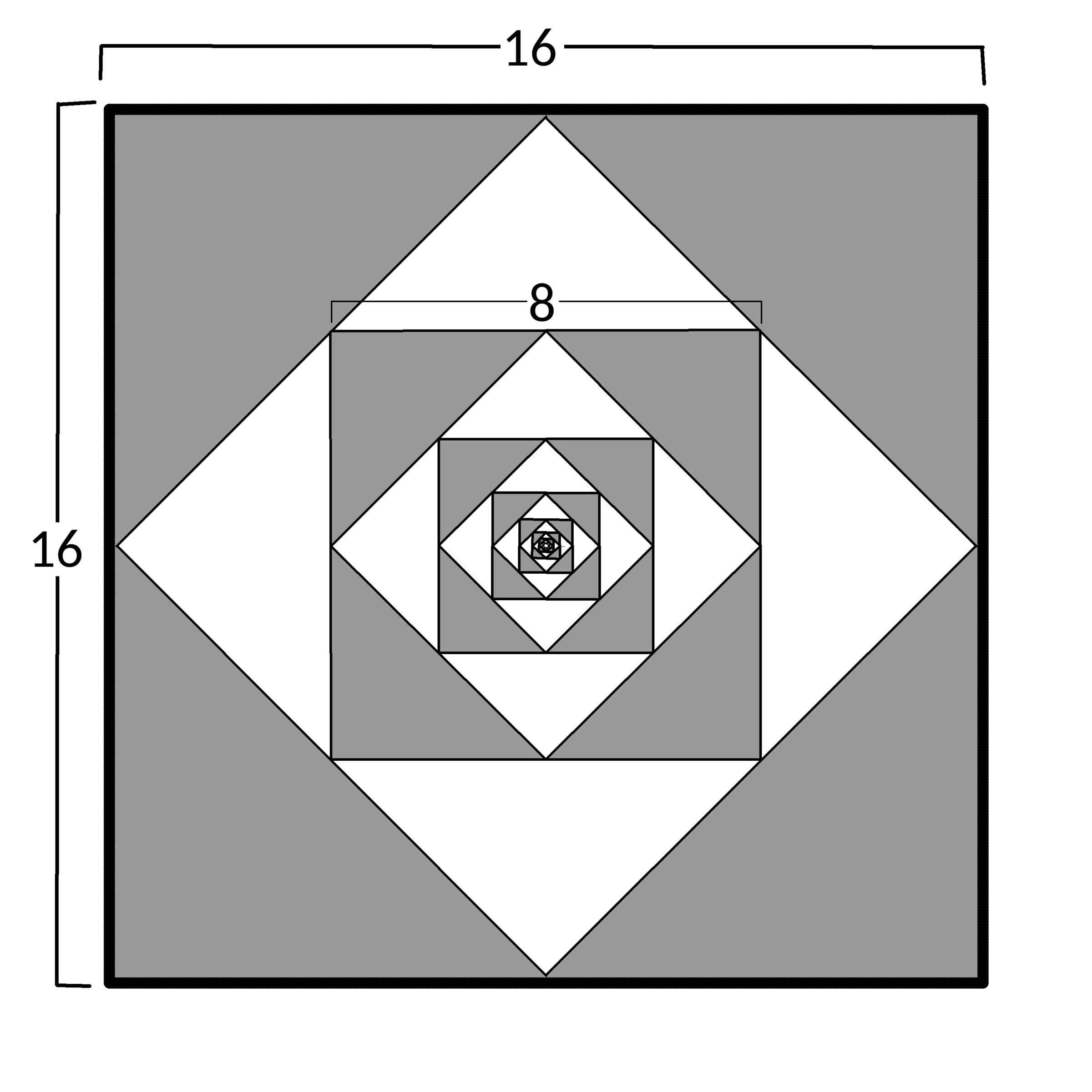
The answer is 259.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
The area of the shaded region can be found by calculating ∑ n = 0 ∞ 4 n 1 2 8 . The area of the non-shaded region is ∑ n = 0 ∞ 4 n 6 4 . Thus ∑ n = 0 ∞ 4 n 1 2 8 − ∑ n = 0 ∞ 4 n 6 4 = 3 2 5 6 a + b = 2 5 6 + 3 = 2 5 9
Area of the 4 triangles is half the area of the square for all squares and area of inner square is half the area of the outer square
So S h a d e d − U n s h a d e d = A ( 2 1 − 2 1 ∗ 2 1 + 2 1 ∗ 2 1 ∗ 2 1 − 2 1 ∗ 2 1 ∗ 2 1 ∗ 2 1 . . . where A = 2 5 6 is the are of outermost square.
This is a G.P with common ratio − 2 1 and its sum can be calculated to be 3 A
Thus our answer is 256+3=259