Superposition is the Key

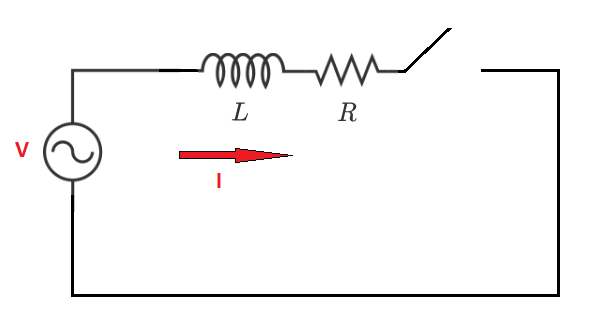
A series $RL$ circuit is excited by an AC voltage source with angular frequency $\omega = \frac{2 \pi}{T}$ . The resistance and inductance values are such that $\frac{\omega L}{R} > 10$ . The source voltage expression is $V(t) = 100 \sin(\omega t)$ . There is a switch in series with the resistor and inductor. Prior to switch closing, the inductor current is zero. The switch closes at time $t = 0$ .

$Q = \int_0^{N T} I(t) \, dt$

The quantity $Q$ is defined as above, where $I(t)$ is the instantaneous current in the circuit (flowing from source to load), and $N$ is a positive integer (greater than zero).

Which statement is correct?

Hints:

1. At $t = 0$ , what is the AC (sinusoidal) portion of the current doing?
2. At $t = 0$ , what is the current through the inductor?