Supporting a Circle

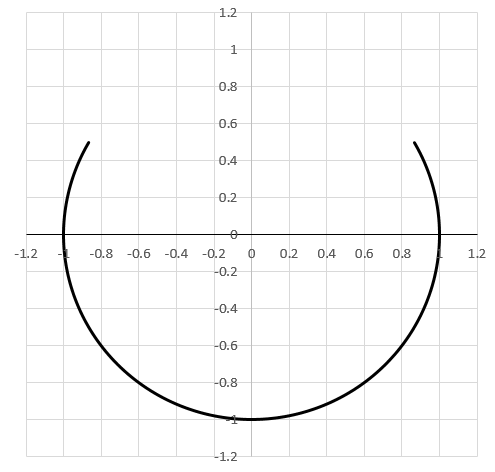
A wire is in the shape of a unit circle centered on the origin, with the portion from $\theta = 30^\circ$ to $\theta = 150^\circ$ missing (see image). The wire's mass is $1 \, \text{kg}$ , and gravity is $10 \, \text{m/s}^2$ in the $-y$ direction.

A force is applied normally to the curve at all points on the curve, such that each unit of curve length experiences an infinitesimal force of the same magnitude:

$\large\frac{d \, |F|}{d \ell} = \alpha{}$

In the above equation, the units on $\alpha$ are $\text{N/m}$ . If the applied force keeps the wire in stasis, what is the value of $\alpha$ ?

Note: My intent is to describe a constant linear pressure over the curve length