Surely It Could Fit

Geometry Level 1

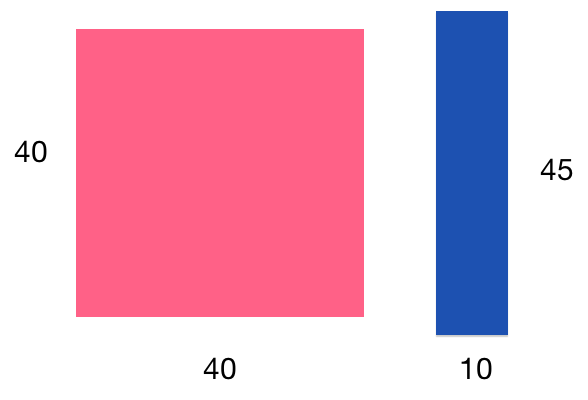
In a square of side length 40, can we fit a $10 \times 45$ rectangle?

Note: You cannot fold / break the rectangle or the square.

Yes No

3 solutions

Calvin Lin Staff
Apr 2, 2016

We can, but just barely!

How can we determine the general solution?
Chew-Seong Cheong claims that in a square of side length $s$ , we can fit a $l \times h$ rectangle where $l > s > h$ if $\sqrt{2} s \geq l + h$ .

$\sqrt{2} a-b \ge c$ , where $a$ , $b$ and $c$ are the side lengths of the square, the long side and short side of the rectangle respectively.

Chew-Seong Cheong - 5 years, 2 months ago

Can you post your solution sharing this result?

Calvin Lin Staff - 5 years, 2 months ago

Shriniketan Ruppa
Sep 10, 2020

The question says "Surely It Could Fit" so the answer is yes. XD

yup! shriniketan

SRIJAN Singh - 9 months ago

Dann Boyer
Dec 4, 2017

Using 10 as the hypotenuse of a right triangle with two 45 degree angles.

a^2 + b^2 = c^2 or in this case 2a^2 = c^2

2a^2 = 100

(2a^2)/2 = 50

a = sqrt 50 approx 7.071

40 - 7.7071 = approx 32.929

This becomes the length of side a and b of a new triangle calculate the hypotenuse.

2 X 32.929^2 = c^2

c = approx 46.569

46.569 > 45, therefore, Yes a 10 unit by 45 unit rectangle can fit inside a 40 unit square.

Surely It Could Fit

3 solutions

0 pending reports