Surface area
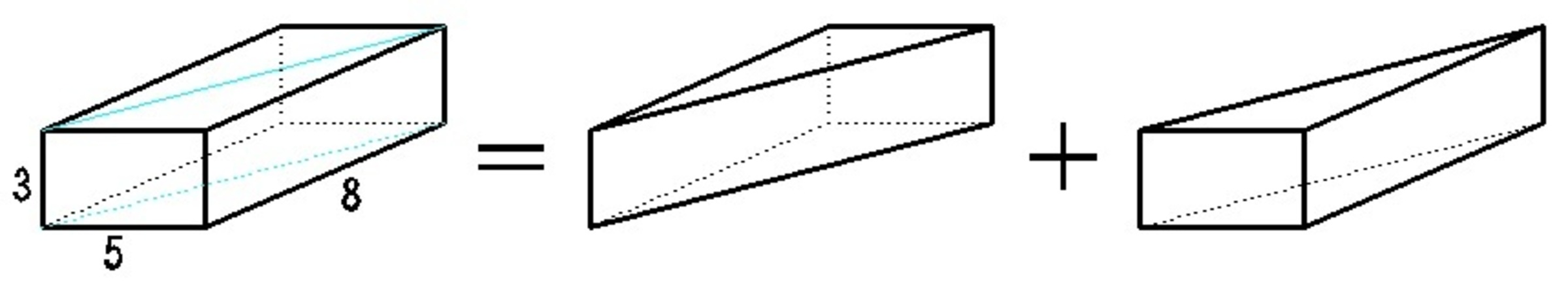 A wood in the form of a rectangular parallelepiped was cut into
equal pieces as shown in the figure. What is the total surface area of the
cuts?
A wood in the form of a rectangular parallelepiped was cut into
equal pieces as shown in the figure. What is the total surface area of the
cuts?
Notes:
-
All units in the figure are in meters.
-
Submit your answer in square meters rounded to decimal place.
The answer is 214.6.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Solving for the length of the diagonal
x = 8 2 + 5 2 = 8 9
Method 1.
Compute the surface area of 1 cut then multiply it by 2 .
A = 3 ( 5 ) + 3 ( 8 ) + 3 8 9 + 2 ( 2 1 ) ( 5 ) ( 8 ) = 1 0 7 . 3
2 A = 2 ( 1 0 7 . 3 ) = 2 1 4 . 6 m 2
Method 2.
Compute the area of the rectangular parallelepiped then add twice the area of the cutting plane.
A = 2 ( 3 ) ( 5 ) + 2 ( 5 ) ( 8 ) + 2 ( 3 ) ( 8 ) + 2 ( 3 ) ( 8 9 ) = 2 1 4 . 6 m 2