Drawing In The Argand Plane

Partial Solution:

The first equation represents a circle with radius Sqrt(2) centered at (-1,-1)

The second equation is a circle of radius 3 centered at the origin.

Clearly, they do not touch each other, which is why there are no solutions

Let be $z = a + ib$ , so we have:

$z + 1 + i = (a + 1) + (b + 1)i$

$|z| = \sqrt{a^2 + b^2} \Rightarrow \sqrt{a^2 + b^2} = 3$

$|z + 1 + i| = \sqrt{(a + 1)^2 + (b + 1)^2} \Rightarrow \sqrt{(a + 1)^2 + (b + 1)^2} = \sqrt{2}$

Raising both equations to the square power we obtain:

$a^2 + b^2 = 9$

$(a + 1)^2 + (b + 1)^2 = 2$

Let's develop the second equation:

$a^2 + 2a + 1 + b^2 + 2b + 1 = 2 \Rightarrow a^2 + 2a + b^2 + 2b = 0$

But the first equation says $a^2 + b^2 = 9$ , so:

$a^2 + 2a + b^2 + 2b = 0 \Rightarrow 9 + 2a + 2b = 0 \Rightarrow (a + b) = -\frac {9}{2}$

being $(a + b)^2 = a^2 + b^2 + 2ab$ we obviously have that $a^2 + b^2 = (a + b)^2 - 2ab$

so:

$(-\frac {9}{2})^2 - 2ab = 9 \Rightarrow 81/4 - 2ab = 9 \Rightarrow ab = 45/8$

So we can build a new equation:

$x^2 + (a + b)x + ab = 0 \Rightarrow x^2 -\frac {9}{2}x + \frac{45}{8}=0$

that has no solutions, being $\Delta=\frac {81}{4} - [4\times \frac{45}{8}] = \frac {81}{4} - \frac{45}{2}=-\frac {9}{4}$

Using Triangular Inequality

$|z|-|i+1| \le |z+1+i| \le |z|+|1+i|$

Also $|z|=3$

$3- \sqrt2 \le |z+1+i| \le 3+\sqrt2$

But $\sqrt2$ does not lie in above range .Hence no solutions

We can solve the two equations considering the complex number $z$ as $z = x + iy$ .

It is a known fact that $|z| = \sqrt{x^2 + y^2}$

The given equations are:

1. $|z| = 3$
2. $|z + 1 + i| = \sqrt{2}$

So equation $(1)$ becomes

$\sqrt{x^2 + y^2} = 3$

$\Rightarrow x^2 + y^2 = 9$

which is the equation of a circle of radius 3 units centered at the origin.

And equation $(2)$ becomes

$|x + iy + 1 + i| = \sqrt{2}$

$\Rightarrow |(x+1) + i(y + 1)| = \sqrt{2}$

$\Rightarrow \sqrt{(x+1)^2 +(y+1)^2} = \sqrt{2}$

$\Rightarrow (x+1)^2 + (y+1)^2 = 2$

which is the equation of a circle of radius $\sqrt{2}$ units centered at $(-1,-1)$ .

For understanding how we get to know the radius and center of the circle, refer Equation of a Circle

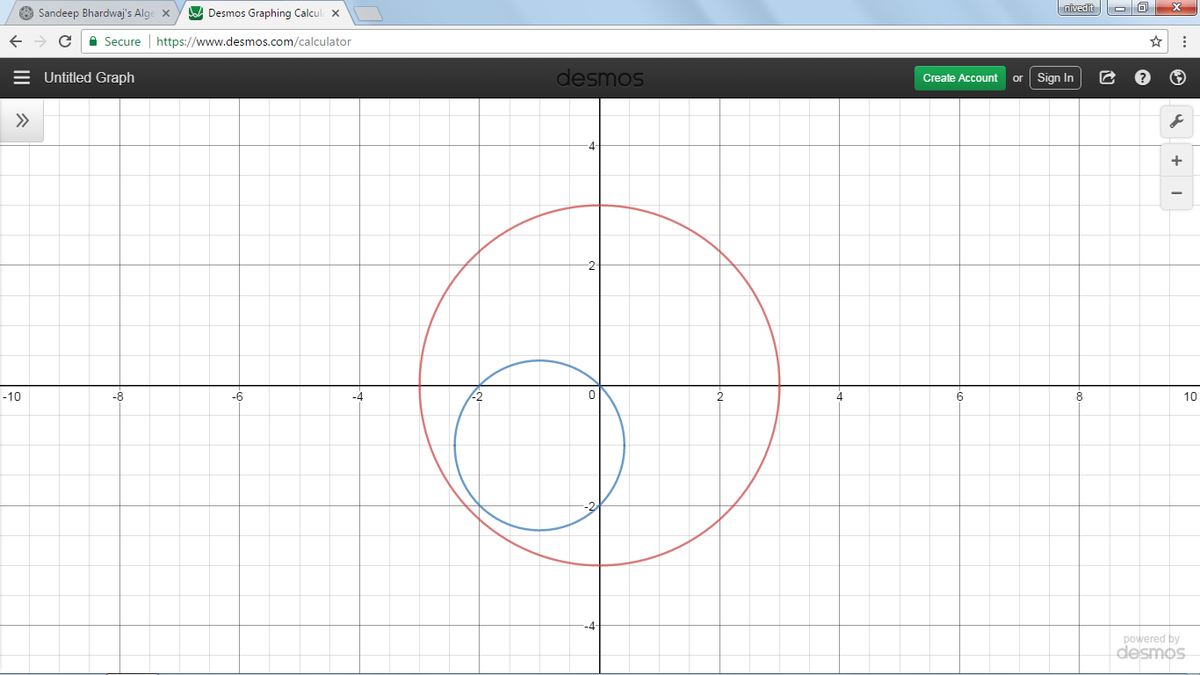
When the graphs of these two equations are plotted in the $xy$ plane they look like this : -

The blue circle is the graph of equation $(1)$ and the red circle is the graph of equation $(2)$

We can clearly see that they do not intersect anywhere in their domain, which means there is no coordinate $(x,y)$ that satisfies both the equations simultaneously . Hence the two equations have no common solution.

For the more rigorous algebraic solution to this answer, you can refer the answer given by Giorgio de Fornasari.

Since |z| = 3, z must be -3 or 3. From this the two equations can be simplified to be |4 + i| = (2)^(1/2) and |-2 + i| = (2)^(1/2). We know that a^2 + b^2 = |a + bi|^2, so 16 + 1 = |4 + i|^2, so (17)^(1/2) = |4+ 1|, since (17)^(1/2) does not equal (2)^(1/2), this equation is wrong. Next |-2 + i| = (-2)^2 + (1)^2 = 4 + 1 = 5, so (5)^(1/2) = |-2 + i|. Since (5)^(1/2) does not equal (2)^(1/2) this equation does not work either. Since both possible options for these equations have been exhausted there is no solution.