Take Off on "Pretty Narrow Alley"

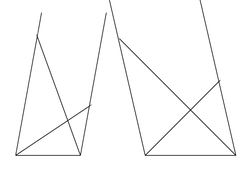Two ladders of different lengths cross in a narrow alley (see graphic below). The rungs of the ladders are spaced a foot apart, from end to end, and the alley is an integer number of feet wide. The ladders cross exactly where there are rungs, i.e., integer number of feet from intersection point to ends of ladders. If no ladder is greater than 30 feet in length, there is ONE unique solution for the width of the alley.

However, if the walls on the sides of the alley are parallelly slanted from the vertical, how many solutions can be obtained?