Adventures in Parallel Dimension #1729 I
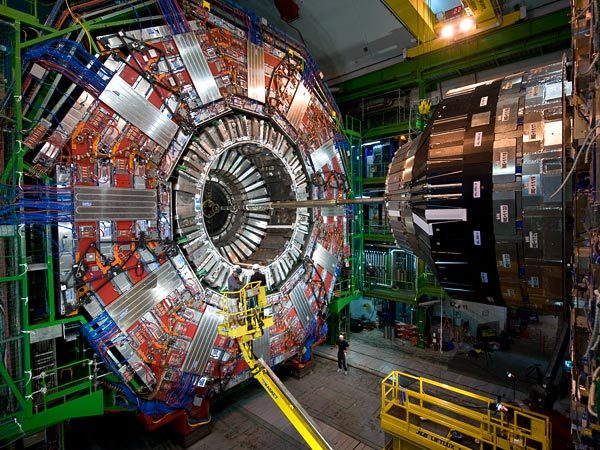
In parallel dimension #1729, CALVIN National Laboratories has discovered a new unstable sub-atomic particle, the Biggs Hoson, which resulted from a high-energy collision between two stable sub-atomic particles in a collision chamber similar to the one at CERN (pictured below).
Thanks to parallel dimensions #1729's awesome technology, they were able to trace the path of the Biggs Hoson after it was born and found that the arc length of its trajectory was exactly meter before decaying, and that its lifespan at rest was exactly seconds.
If is the average speed of the Biggs Hoson after the collision, what is the value of rounded to 3 decimal places?
Details and Assumptions:
- is the speed of light, which also happens to be in parallel dimension #1729.
- Light speed is still the universal speed limit. Nothing can travel faster than a photon, and it always travels at relative to any intertial observer.
The answer is 0.958.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Relevant wiki: Special Relativity
This problem deals with the Lorentz Contraction and Time Dilation concepts from Special Relativity :
The Biggs Hoson's lifespan at rest, t 0 , is the same thing as the lifespan of the particle traveling at v 0 from the particle's frame of reference. Similarly, the measured distance d that the Biggs Hoson travels is from the frame of the scientists taking the data.
Let's define t 0 ′ to be the lifespan of the Biggs Hoson from the frame of the scientists and d ′ to be the distance traveled by the Biggs Hoson during its lifespan in the frame of the particle.
Then t 0 ′ = t 0 γ and d ′ = γ d for γ = c 2 − v 2 c .
If we calculate average velocity in the frame of the scientists then:
v 0 = t 0 ′ d = t 0 γ d = t 0 c d c 2 − v 0 2 ⇒ v 0 2 t 0 2 c 2 = d 2 c 2 − d 2 v 0 2 ⇒ v 0 = c 2 t 0 2 + d 2 d c .
It follows that v 0 c − 1 ∣ t 0 = 1 0 − 9 s , d = 1 m ≈ . 9 5 8 .
Note that it would not matter whose frame we calculate average velocity from, which is to be expected. By doing it from the Biggs Hoson's perspective distance is d ′ and time is t 0 , which would give rise to the exact same equation.