The area is almost whole.

Consider the first quadrants of the two circles. Let $A$ and $B$ be the centers of the two circles, therefore, $AB=1$ , and $AC=5$ and $BD=5$ be their radius on the $x$ -axis. Point $P$ is where the two circles intersect and $O$ be the mid-point of $AB$ . By symmetry, $OP$ is perpendicular to $AB$ .

The shaded area $[PCD]$ ( $[\cdot]$ denotes the area) is given by:

$\begin{aligned} [PCD] & = {\color{#3D99F6} [OPD]} - \color{#D61F06} [OPC] \\ & = {\color{#3D99F6} [BPD]+[OPB]} - \color{#D61F06} ([APC]-[OPA]) \\ & = \frac {5^2(\pi + \theta)}2 + \frac {5^2\sin \theta \cos \theta}2 - \frac {5^2(\pi - \theta)}2 + \frac {5^2\sin \theta \cos \theta}2 \\ & = 25(\theta + \sin \theta \cos \theta) & \small \color{#3D99F6} \text{Note that } \sin \theta = \frac {\frac 12}5 = 0.1 \\ & = 25\left(\sin^{-1} 0.1 + 0.1 \sqrt{1-0.1^2}\right) \\ \implies A & = 4\times 25\left(\sin^{-1} 0.1 + 0.1 \sqrt{1-0.1^2}\right) \approx 19.967 \\ \epsilon & = \lceil A \rceil - A \approx 20 - 19.967 = 0.033 \\ \implies \frac 1\epsilon & = \frac 1{0.033} \approx \boxed{30} \end{aligned}$

I'll commit a venial sin and post a calculus solution to a geometry problem. Say $R = 5$ and $D = 1$ . The two circles are:

$\large{x^2 + y^2 = R^2 \\ (x-D)^2 + y^2 = R^2 \\ y_1 = \sqrt{R^2 - x^2} \\ y_2 = \sqrt{R^2 - (x-D)^2}}$

It is fairly easy to determine that the intersection between the two circles occurs at $x = \frac{D}{2}$ . Half of the shaded area is therefore equal to:

$\large{\frac{A}{2} = \int_{D/2}^R \, 2 (y_2 - y_1) \, dx + \int_R^{R + D} \, 2 \, y_2 \, dx}$

This yields:

$\large{A \approx 19.9666165 \\ \frac{1}{\epsilon} \approx 29.9549}$

Below is a picture showing the centers of the circles and the place where the regions almost overlap. It is turned sideways from the original drawing above. The centers of the circles are $A$ and $B$ so $AB=1$ and $AD=5$

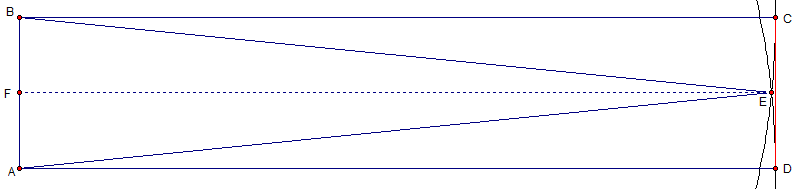
The error is the area of four copies a little almost-triangle $CED$ but with two curved sides. The area of this triangle can found by taking rectangle $ABCD$ and subtracting triangle $AEB$ and the two sectors $CBE$ and $DAE$ .

$AB=1, AD=5$ so the area of rectangle $ABCD$ is $5$

$EF$ is the leg of triangle $AFE$ . $0.5^{2}+EF^{2}=5^{2}$ so $EF=\frac{3}{2}\sqrt{11}$ . Area of triangle $AEB=\frac{3}{4}\cdot \sqrt{11}$

The sectors have central angle $\sin ^{-1} (1/10)$ and so each has an area $\pi \cdot 5^{2} \cdot \frac{\sin ^{-1} (1/10)}{360^{\circ}}$

So the area of the one almost-triangle is

$5 - 2\cdot \pi \cdot 25 \cdot \frac{\sin ^{-1}(1/10)}{360^{\circ}}-\frac{3}{4} \cdot \sqrt{11} \approx 0.0083458782$

The error is four of these so $\epsilon = 0.0333835128$ and $\frac{1}{\epsilon}=29.95490636$ which is very close to the whole number $\boxed{30}$

$Total~~ area~~ of~~ two~~ blue~~ region\\ =2*\{Segment(APB)~-~Segment(AQB)\} \\ =2*\{(\pi-2*Cos^{-1} (.1))*25+\sqrt{24.75}\}=19.9666.....\approx 20.\\ \therefore~\epsilon=20 - 19.9666....\\ \dfrac 1 \epsilon=29.9549.\\ The~ required~ answer=\Huge~~\color{#D61F06}{30}.$