The block has unreliable power, it will not move...
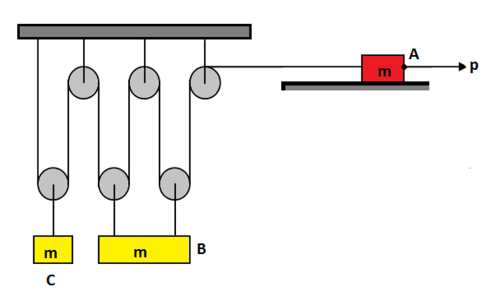Three blocks
each of mass
are arranged in pulley string system as shown in figure. All pulleys are massless and frictionless , while all strings are light and inextensible. Coefficient of kinetic friction between block
and horizontal surface is equal to
and force
acts on it. The value of
for which block
does not move, is equal to?
The answer is 5.000.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
We use Result 1 (see figure) repeatedly to get relation between acceleration of masses A and B .
Result 2 : As the pulleys are massless F N E T on pulleys is equal to 0.
We use Result 2 repeatedly to get relation between tension in string attached next to masses A and B .
Now we apply newton's second law of motion for masses A , B and C .
P − μ m g − T = 4 m a 4 T − m g = m a 2 T = m g
Solving above 3 equations simultaneously we get m g p = 5 .