The Case Of The Spinning Spaceship

Classical Mechanics Level 2

The extraterrestrial Federation of Goboria wishes to build an interstellar spaceship to explore their galaxy. They contact Engineer Eevis, who draws up the following plans:

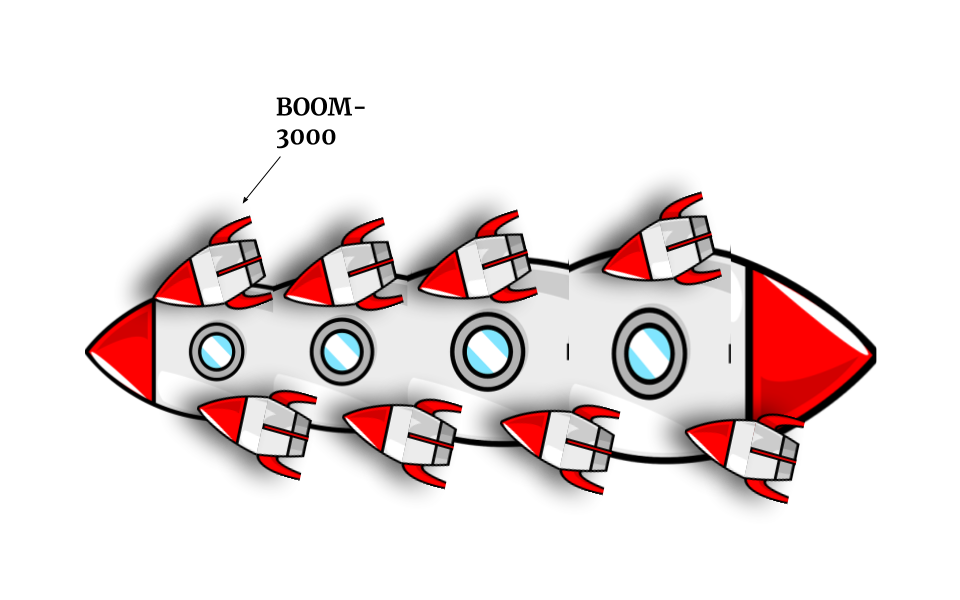
However, during the test runs, it is found that the ship has an alarming tendency to spin! (Much to the dismay of the crewmembers' guts.) To find a solution, the Federation of Goboria contacts four of its greatest scientific minds: Physicists Saugool, Amon, Pauto, and Mondrok.

"The BOOM - $3000$ antimatter engines are so powerful, they are making the spaceship wobble like jelly," Amon declares. "Wobbling downwards, I mean. Make the spaceship's structure more rigid."

"No!" Mondrok retorts. "The answer lies in the vibrations of the engines. They are making the spaceship spin."

"Why, the answer is elementary," Pauto says, smiling. "The turning effect, uh, moment of the engines does not cancel for both sides."

"Pauto, your answer makes no sense unless the mean momentum of the exhaust is not equal for the engines," Saugool comments. "I am convinced that the magnetic field generated by the exhaust is deviating the ship."

Who is correct?

Details and assumptions

Each engine is equally distanced from the engine directly in front and/or behind, and the centerline of the ship.
The angle formed by the ship's centreline and the engines is equivalent for all engines, and is greater than 0.
The first and last engines are placed directly on the bow and stern, respectively.
Each engine provides exactly the same amount of thrust.
The BOOM - $3000$ efficiently converts all of its energy into thrust in the direction of the engines. (I know this doesn't happen in real life, but assume that the discrepancy is so small that no feasible instrument could effectively measure it.)
The ship has infinitely many degrees of rotational symmetry around its central axis, from bow to stern.

The next problem can be found here .

Amon No one is correct. Pauto Mondrok Saugo

1 solution

Andrei Li
Aug 15, 2018

The BOOM - $3000$ efficiently converts all of its fuel into forward thrust, which allow us to discard Mondrok's theory. Amon's theory can be eliminated by intuition: 'wobbling' is vibration, which would not cause the ship to spin. Antimatter and matter reactions only produce gamma radiation: thus, Saugool's theory is eliminated. This leaves us with only Pauto's theory, that the "twisting force" of the engines is not balanced out. And this is not due to the mean momentum of the exhaust not being parallel to the axis of the ship, as Saugool comments.

To see why Pauto is correct, let us draw a diagram:

We see that the force vector $\mathbf{F}$ can be expressed as two component vectors: $\vec a$ , perpendicular to the distance $d$ from the centreline of the ship, and $\vec b$ , perpendicular to the ship's central axis.

Now, we apply Archimede's Law Of The Lever :

The turning effect , or moment , on a lever, is equal to the force multiplied by the perpendicular distance from the fulcrum to the point of application . For example, a mass placed on the end of a lever a distance $d$ from the fulcrum is balanced by half the mass applied at a point $2d$ from the fulcrum, on the opposite arm. (The acceleration is provided by gravity.)

For all of the engines, the moment $m$ of $\vec a$ is $m=4d\vec a-4d\vec a=0$ , as there are four engines on each side applying an equal force component $\vec a$ , all equally distanced from the centreline. Note that moments are positive for engines 'on top' of the ship (counterclockwise on diagram), and negative for engines 'on the bottom' of the ship (clockwise on diagram).

To calculate the moment, set $d_{engine}$ equal to the distance between consecutive engines. Then, $0, d_{engine}, 2d_{engine}...nd_{engine}$ is the distance between the $n$ th oar and the stern ( $d_{engine}=0$ ).

$m=0+d_{engine}\vec b-2d_{engine}\vec b+3d_{engine}\vec b-4d_{engine}\vec b+5d_{engine}\vec b-6d_{engine}\vec b+7d_{engine}\vec b=4d_{engine}\vec b$

As the moment is non-zero, the ship rotates counterclockwise, accounting for the 'spinning motion'. Pauto was correct.

The Case Of The Spinning Spaceship

1 solution

0 pending reports