A Powerful Comparison

$\Large 2^{999} = 2^{979} \times 2^{20} = 2^{979} \times 1024^2 > 1024^2 > 999^2.$

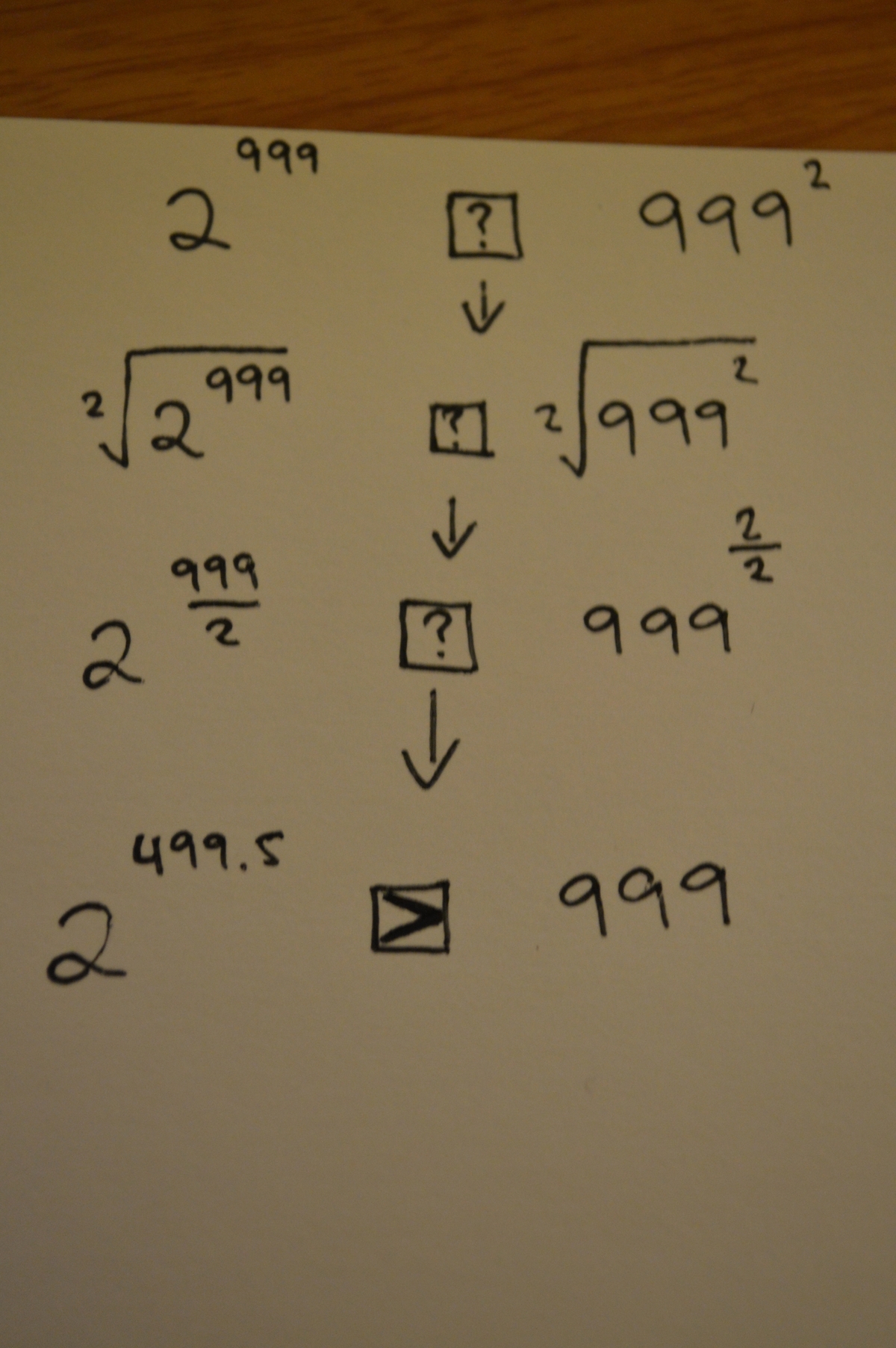
Then you will be able to say (if you have ever powered 2 to anything) that 2^{10} (1024 I believe) itself is larger than 999.

Then 2^{499.5} must be!

Taking logs of both numbers we get $\large{ 2 }^{ 999 }\Rightarrow \log { { 2 }^{ 999 } } = 999\log { 2 } \approx 1000\times 0.3=\boxed { 300 } \\ \large { 999 }^{ 2 }\Rightarrow \log { { 999 }^{ 2 } } = 2\log { 999 } \approx 2\times 3= \boxed { 6 }$

It is clear that ${ 2 }^{ 999 }$ will be larger than ${ 999 }^{ 2 }$ .

(999 )(999)=(6 digit number) .
(2^10)
(2^10) (2^979)=(1024) (1024) (2^479)=(8 digit number) (2^479).
8 digit number > 6 digit number.

power increases the value very rapidly

such as $999^2=998001$

and , $2^{20}=1048576$

so, $2^{999}> 999^2$

Since both number base ie, 2 and 999, positive, we can use logarithm test. So clearly, 999ln2 (999x0.3=300 approx) is greater than 2ln999 (approx. 2x3=6) . Hence 2^999 is greater.

This problem can be easily be solved by logic as well as by binomial theorem

Note That: 2^999 = (2^20) (2^979) =(2^10)^2 (2^297)=2^297 (1024)^2 > 1024^2 > 999^2 From the above inequality, obviously that: 2^999 > 999^2.

squaring both terms or 2^999/2 and 999^2/2 becomes 2^449.5 and 999 respectively. Now the question is how many times would you raised up the value of 2 to atleast equal the value 999. Or in eqtn 2^x=999, solving for x= ln(999)/ln2=9.96434 or we can say that 2^10>999.....far less when 2 is raised up to 499.5.

My maths is rather rusty after all these years. I manage to get the answer right but my reasoning might be wrong. I reasoned that 2^9 > 9^2 and so 2^999 must be bigger than 999^2. Correct me if I am wrong.

I just guessed😀😏

999^2 would give you a 6 digit number because the digits are always doubled when we square . Then you get 2^14 as 4102 and 2^999 is definitely going to be over 6 digits.

because power increases the value rapidly

1000x1000 is greater than 999^2 and equals 1 million. 32 bit computers cannot address memory beyond 4gb so 2^32 is approx 4 billion. So 2^32 is approx 4000 times greater than 1000^2 therefore 2^999 is hugely bigger than 999^2. Not very mathematical I know but for me was the easiest way to figure it out

[ln(999^2)= 2ln (999)] < [ln(2^999)=999 ln(2)]; Note: 1) ln(999) it's a small number. 2) if ln(x)<ln(y) => x<y

2^999 = 999 * lg2 and lg 2 is between 1 and 2, so that makes it certainly more than 999; 999^2 = 2 * lg 999 and lg 1000 = 3, so lg 999 is certainly less than 3 and that makes it less than 6. Conclusion, 999>6.

Thought this might be useful: the number of digits in the powers of 2 increase when the power ends with 0, 4, 7 e.g. 2^0 = 1(1 digit). 2^4 = 16 (2 digits). 2^7 = 128 (3 digits). 2^10 = 1024 (4 digits) etc. Going by this, there would already be 30 digits in 2^99, and about 300 digits in 2^999. 999^2 on the other hand, = 9^2 * 111^2 = 81 * 12321 which can never be anywhere close to 300 digits

Under speculation with the exponential trend this was an easy question.

Layman's solution... Well, 1000 squared is 1,000,000. 2 to the power of 999 is going to be way more than 1,000,000.

First, I rounded up to make it easy to do in my head, 1000X1000=1,000,000. (6 zeros) 10to 999 would have 999 zeroes, so, even after you divided by 5 to get back to the original 2, there are still WAY more than 6 zeros on the end of the answer??

it can be solved by log also

1000 times 1000 with produce a figure containing 7 digits; so 999 times 999 cannot produce a figure exceeing 7 digits. 2 raised to the power of 999 will result in a figure far exceeding 7 digits.

You just have to use common logic, of course it's 2^999, cause it's the one that at first seems smaller

2^10 = 1024; 2^20 = 1024 x 1024; 900^2 = 810 000; So, 2^20 > 900^2; and 2^999 > 999^2

999 is nearer to 1000 so square of 999 will be less than 2 power 999

I solved it focusing the problem in the divisors of each number. If we compare the divisors, we can prove that 2^999 is greater than 999^2.

Can be solved by binomial theorem

Obviously that 2^999 is bigger than 999^2. In one case you have 999 calculations of 2 2, while in the other 999^2 you have only one of 999 999.

In general,

$a^{b} > b^{a}$ for a< b since 2 < 999, then, $2^{999} > 999^{2}$

2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x 2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2 x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2x2>999x999

I counted, There was really 999 2x. I was about to write down what 2^999 was, but soon I figured that it would be a 300 digits number.... and gave up.

Log2^999>log999^2, log to the base 2.

exponents get wild really fast, so that's probably bigger than just something squared. :)

You can round it 2^999 ~2^1000 and 999 ^2 is ~1000*1000.

if we go on doubling 2, beyond a point it is huge compared to multiplying 999 only 2 times

Can't we use the 'no. of possibilities or cyclicity?

Let's assume 999^2 as 1000^2. We know from simple multiplication that 1000^2 is 1 million. But we also know that even 2^27> 1000000. And it's obvious that 1000^2> 999^2. Meaning 2^999>999^2.

log2 (2^999) = 999

log2 (999^2) = 2log2 (999)

log2 999

2^10 = 1024

let's assume that log2 999 = 9

so 2log2 (999) = 18

999 > 18

so

2^999 > 999^2

Do you even need a solution for this ? especially, when there is a calculator.

(2powe10) = 1024 99 to the power of 1024 obviously greater than 2 power to the 999.

it can be done with simple logic i.e 2^999 will consist 2 ,999 times whereas 999^2 will consist 999 , 2 times so we can easily say that 2^999 is the correct ans.

Knowing properties of exponents and rewriting the fractions using estimates is how I solved it.

To show that 2^999 is larger than 999^2 , simply take log base 2 or log base 999 of both sides.

After completing that, I was left with

999>2log_2(999)

If you understand logs, simply looking at this makes it obvious that the LHS is much larger, but more simplification makes it more clear.

999/2 (~500) > log_2(999)

You know that 500 is larger than log 2 (999), because log 2(1024) is larger than log_2(999) and it is only equal to 10.

999/2 is clearly much larger than 10.

This is an eyeballer when you simplify.