The Great Divide

Geometry Level 4

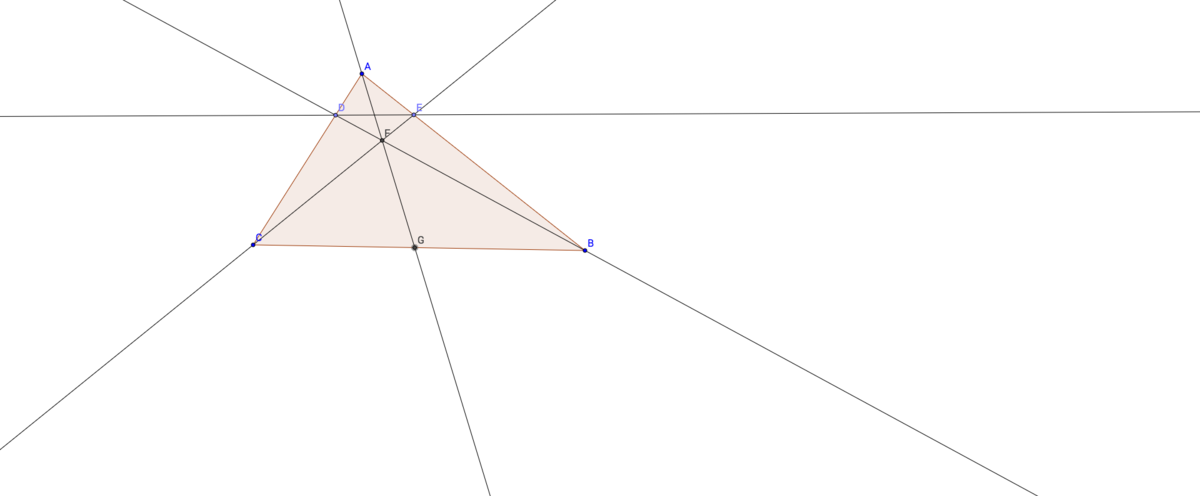
You have a triangle ABC and you draw a line from a point D on AC such that it is parallel to BC and it meets AB at E. Join EC and BD and these lines meet at F. AF meets BC at G.

If G C B C = a b \frac { GC }{ BC } =\frac { a }{ b } , find a + b a+b .


The answer is 3.

View wiki
Vishnu C by vishnu c , 22, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Vishnu C
May 11, 2015

Ceva's theorem is applicable here as AG, CD and BE are concurrent.

S o y o u h a v e A D D C = A E E C b y s i m i l a r t r i a n g l e l o g i c a n d C G × B E × A D = D C × G B × E A , u s i n g C e v a ′ s t h e o r e m . W e g e t , a f t e r c a n c e l l a t i o n , C G = G B . S o , a = 1 a n d b = 2 t o g i v e a + b = 3. So\quad you\quad have\quad \frac { AD }{ DC } =\frac { AE }{ EC } \quad by\quad similar\quad triangle\quad logic\\ and\quad CG\times BE\times AD=DC\times GB\times EA,\quad using\quad Ceva's\quad theorem.\\ \\ We\quad get,\quad after\quad cancellation,\quad CG=GB.\quad So,\\ a=1\quad and\quad b=2\quad to\quad give\quad a+b=\boxed { 3. } \

4 Helpful 0 Interesting 0 Brilliant 0 Confused
Li Yuelin
May 11, 2015

Assume that D and E are the midpoints of AB and AC respectively. Thus F is the centroid of ABC. It therefore follows that AG is a median, G is the midpoint of BC, and a/b = 1/2. 1+2 = 3

0 Helpful 0 Interesting 0 Brilliant 0 Confused

Can you prove it for a general DE? D or E need not be the midpoint.

vishnu c - 6 years, 1 month ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...