The Holey Cube
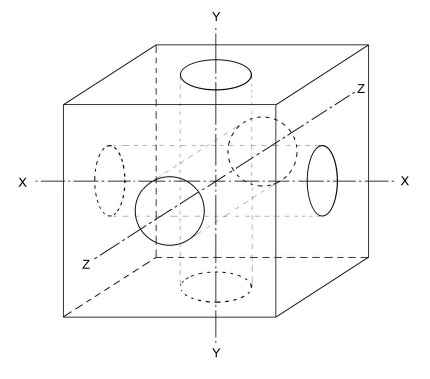A cube of length 10 cm is drilled through the centre of three faces (as pictured above). If the radius of the circular drill bit is 2.5 cm, what is the volume of this shape in cubic centimetres?
If you get stuck, try this simpler problem .
The answer is 587.73.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
The volume of the material removed from the 10 x 10 x 10 (or c x c x c for the general case) cube is based on the formula:
6 x pi x r^2 x (c - 2r)/2 + 4r^3[3pi/4 + sqrt(2)/2 - (3pi.sqrt(2))/8 + 1/2]
where c, is the length of each side of the cube; r, is radius of the drilled hole;
For c = 10 cm and r = 2.5 cm, the volume of the removed material is 413.1005719 cm^3 and so the remaining solid with 3 intersecting holes is 1000 - 413.1005719 = 586.8994281 cm^3. This does not quite match the answer held on site but I am sure my answer is the correct one.