The inner circle
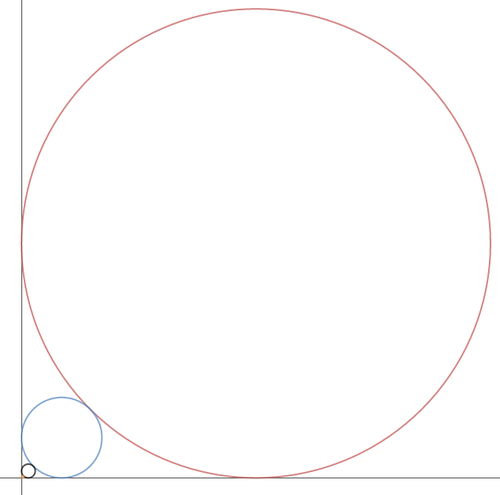 A circle of radius
is drawn tangent to the
and
axes. This is circle #1, shown above as the largest, red circle.
A circle of radius
is drawn tangent to the
and
axes. This is circle #1, shown above as the largest, red circle.
Circle #2 is a smaller circle drawn tangent to the and axes and to circle #1.
Circle #3 is an even smaller circle tangent to the and axes, and to circle #2.
In general, circle #N is drawn tangent to the and axes and to circle #(N-1).
Which decimal is closest to the ratio of the area of circle #101 to the area of circle #100?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
First, find the radius of circle #2 in terms of a. The distance from the origin to the point of tangency between circle #2 and circle #1 is
d = ( 2 − 1 ) a .
This distance is related to the radius of circle #2 by
d = r + 2 r ,
giving us that
r = 2 + 1 d .
In turn, that gives us a relationship between r and a :
r = k a
where
k = 2 + 1 2 − 1 .
By inspection, the radii of circles #3 and #2 should have the same ratio of k between them; thus the ratio of the radii of circles #(N+1) and #N should be k , and the ratio of the areas of circles #(N+1) and #N should be k 2 . This is a general formula, so it should hold for circles #101 and #100.
This gives us a value of approximately 0 . 0 2 9 , which is closest to 0 . 0 3 .