The Limiting limit

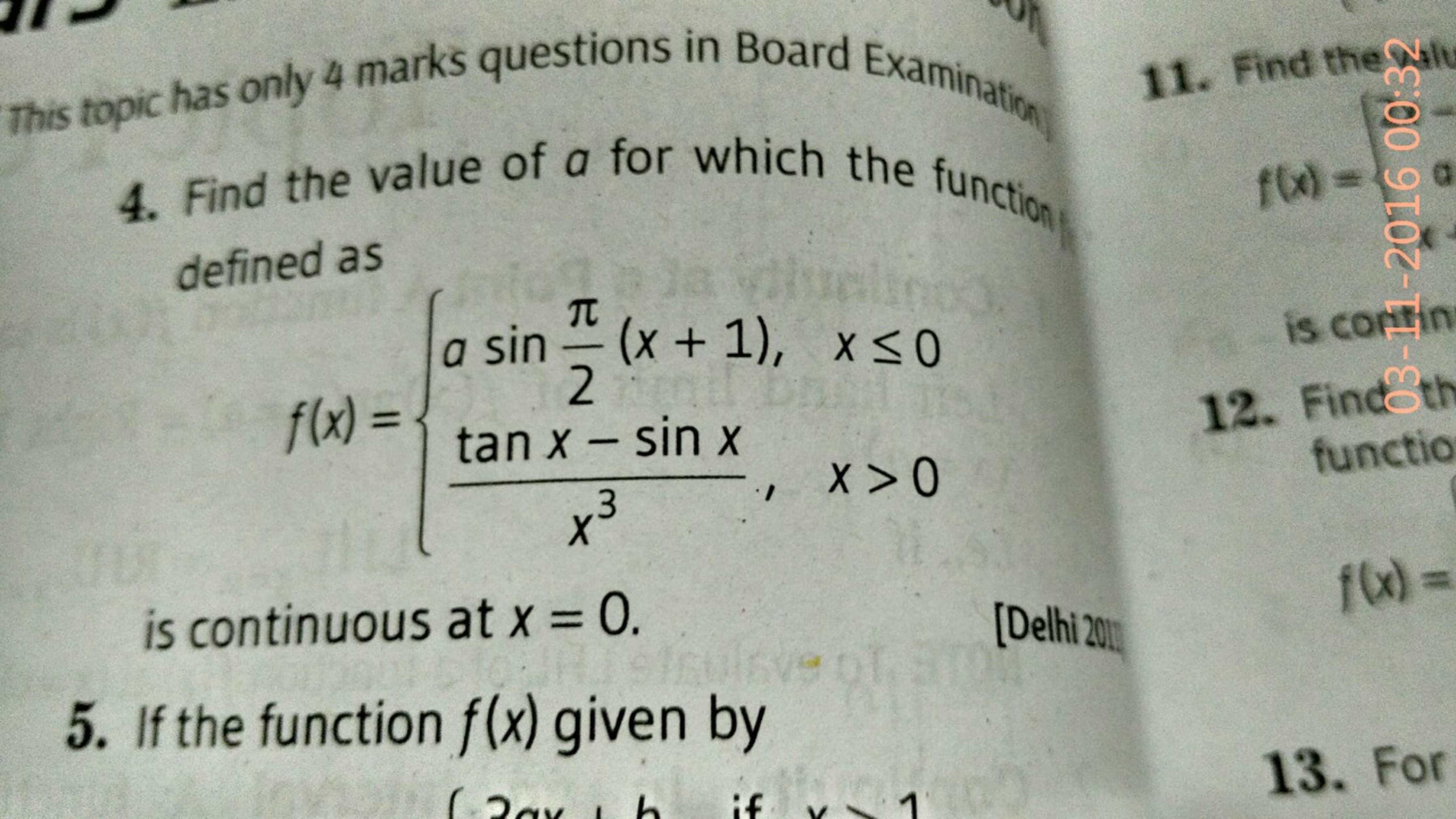
Answer the question number 4. Try not to use L'Hôpital's Rule P.S. I have written a doubt in solution section please help me with it if you can.

Moderator's edit :

Find the value of $\alpha$ for wihch the functiion $f(x)$ is defined as

$f(x) = \begin{cases} \alpha \sin \dfrac{\pi}2 (x+1) , \qquad x \leq 0 \\ \dfrac{\tan x - \sin x}{x^3} , \qquad x> 0 \end{cases}$

and is continuous at $x=0$ .