The Parabolic Minimum

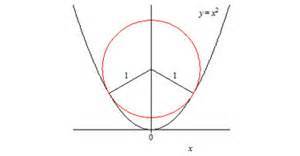
Let's consider $P=(t,t^2), t \in \mathbb{R}$ a generic point on the parabola. The tangent line to $y=x^2$ , passing through $P$ has equation $y_t=mx+q$ , where $m=\frac{dy}{dx}=2x$ . So,

$\displaystyle t^2=2t \cdot t+q \hspace{5pt} \Longrightarrow \hspace{5pt} q=-t^2 \hspace{5pt} \Longrightarrow \hspace{5pt} y_t=2tx-t^2$

Let's call $y_n=m'x+q'$ the normal line to $y_t$ . Since the two lines are prependicular, $m'=-\frac{1}{m}=-\frac{1}{2t}$ . Hence,

$\displaystyle t^2=-\frac{1}{2t} \cdot t+q' \hspace{5pt} \Longrightarrow \hspace{5pt} q'=t^2+\frac{1}{2} \hspace{5pt} \Longrightarrow \hspace{5pt} y_n=-\frac{x}{2t}+t^2+\frac{1}{2}$ .

The point $C$ we get intersecting $y_n$ and $x=0$ is the center of the circle tangent to the two branches of the parabola.

$\begin{cases} \displaystyle y_n=-\frac{x}{2t}+t^2+\frac{1}{2} \\[8pt] \displaystyle x=0 \end{cases} \hspace{5pt} \Longrightarrow \hspace{5pt} \displaystyle C(0,t^2+\frac{1}{2})$

The radius of the circle is $\overline{CP}=1$

$\displaystyle \overline{CP}=\sqrt{(t-0)^2+\bigg(t^2-\bigg(t^2+\frac{1}{2}\bigg)\bigg)}=1 \hspace{5pt} \Longrightarrow \hspace{5pt} t=\pm \frac{\sqrt{3}}{2}$

We truncate the parabola at $h=1+C_y=\frac{3}{2}+t^2=\frac{9}{4}$ . The volume of the sphere is $V_p=\frac{4}{3} \pi$ . The volume $V_p$ of the paraboloid generated by the rotation of the parabola around $y$ can be easily evaluated if we consider the rotation of $y=\sqrt{x}$ around $x$ .

$\displaystyle V_p=\pi \int_{0}^{h} \sqrt{x}^2 dx=\pi \int_{0}^{\frac{9}{4}} x dx=\frac{81\pi}{32}$ .

Eventually $\displaystyle \frac{V_p}{V_s}=\frac{81 \pi}{32} \cdot \frac{3}{4 \pi}=\boxed{1.89844}$