The Pyramid Scheme!

Figure 1 As depicted in figure 1, there are 4 copies of $P$ on the four vertices of $ABCD$ (coloured in cyan) and, among them, 8 other copies of $P$ on every edge of the big tetrahedron. This makes a total of $4+6\times 8=52$ copies of $P$ . Between every two adjacent small tetrahedrons there is a square pyramid with all its edges being unit segments.

In order to calculate the volume of such a pyramid we need to know the length of its height.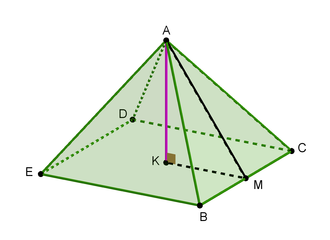 Figure 2 In figure 2, we have $KM=\dfrac{1}{2}$ , $AM=\dfrac{\sqrt{3}}{2}$ , thus, by Pythagorean theorem, $AK=\sqrt{A{{M}^{2}}-K{{M}^{2}}}=\sqrt{{{\left( \dfrac{\sqrt{3}}{2} \right)}^{2}}-{{\left( \dfrac{1}{2} \right)}^{2}}}=\dfrac{1}{\sqrt{2}}$ Hence, ${{V}_{pyramid}}=\dfrac{1}{3}\left[ BCDE \right]\cdot AK=\dfrac{1}{3}\times {{1}^{2}}\times \dfrac{1}{\sqrt{2}}=\dfrac{1}{3\sqrt{2}}$ The volume of the part through which the tetrahedron $P$ passes, is the compound volume of the 52 copies of $P$ plus the volume of the pyramids. On every edge there are 9 of them, but the ones nearest to the vertices of $ABCD$ do overlap, producing a regular octahedron (two pyramids with common base) form which a part is not covered by moving $P$ . This part corresponds to the polyhedron outlined with black edges in figure 3. Its volume is one fourth of the pyramid, hence, near every vertex of $ABCD$ we have a volume of $\left( 2-\dfrac{1}{4} \right){{V}_{pyramid}}=\dfrac{7}{4}{{V}_{pyramid}}$ covered by moving $P$ .

Figure 3

We denote by ${V}'$ the volume of the part inside $ABCD$ through which the tetrahedron $P$ does not pass and by ${{V}_{P}}$ the volume of $P$ .
The similarity ratio of $ABCD$ and $P$ is $r=\dfrac{\text{edge of }ABCD}{\text{edge of }P}=10$ , hence ${V}'={{r}^{3}}{{V}_{P}}\Rightarrow {V}'=1000{{V}_{P}}$ Doing the calculations for the required number, $\begin{aligned} \dfrac{{{V}'}}{{{V}_{p}}} & =\dfrac{{{V}_{ABCD}}-\left\{ 52{{V}_{P}}+\left( 6\times 7+4\times \dfrac{7}{4} \right){{V}_{pyramid}} \right\}}{{{V}_{P}}} \\ & =\dfrac{{{10}^{3}}{{V}_{P}}-\left( 52{{V}_{P}}+49{{V}_{pyramid}} \right)}{{{V}_{P}}} \\ & =\dfrac{948\times \dfrac{{{1}^{3}}}{6\sqrt{2}}-49\times \dfrac{1}{3\sqrt{2}}}{\frac{{{1}^{3}}}{6\sqrt{2}}} \\ & =948-98 \\ & =850 \\ \end{aligned}$ Thus, ${V}'=850{{V}_{P}}$ . The answer is $\boxed{850}$ .