The quadratic with a root of a root of a quadratic
The equation x 2 − a x + 1 = 0 has real solutions, the greater of which is r .
Now, let b be a positive real number such that the greater solution of the equation x 2 − b x + 1 = 0 is r .
If a = 7 9 , then what is b ?
The answer is 9.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
9 solutions
Very nice.
Wait how does solution 2 work? Why is one of them +b?
Log in to reply
The value x is here sqrt(r). So the expression in the brackets on the left side is zero. So the whole product is zero. If you multiply with the other expression you get the equation with factor a and solution r.
In both equations the product of the roots is 1. So the first equation has roots r and r 1 while the second has roots r and r 1 .
Considering sums of roots we have r + r 1 = a and r + r 1 = b
Squaring the second of these equations yields r + r 1 + 2 = b 2 and therefore a + 2 = b 2
Substituting a=79 and taking the positive square root gives b = 9
Forgot the ^2 in the first appearance of b in your third paragraph.
Beautiful, simple solution!
Good and simple solution.
Can you please detail the part where you say that « in both equation products of the roots is 1 » ? Thanks
Log in to reply
It comes from the Viete formulas: in a polynomial equation, the product of the roots equals the coefficient of the zero-degree term divided by the coefficient of highest degree.
Given that r is a root of x 2 − a x + 1 = 0 , therefore, r 2 − a r + 1 = 0 and ⟹ a = r r 2 + 1 . Similarly, given that r is a root of x 2 − b x + 1 = 0 , then
b = r r + 1 = r ( r + 1 ) 2 = r r 2 + 2 r + 1 = a + 2 = 7 9 + 2 = 9 Note that a = r r 2 + 1 and a = 7 9
r 2 − 7 9 r + 1 = 0 ( r 2 + 2 r + 1 ) − 8 1 r = 0 ( r + 1 ) 2 = 8 1 r Since r > 0 , taking square-root on both sides, we get r + 1 = 9 r r 2 − 9 r + 1 = 0 So b = 9
Product of the roots is=1 fir both equations, the sum of the roots give relation b^2-2=79, therefore,
Answer is, b=9
We will use the fact that for a monic quadratic equation the sum of the roots is given by the negative of the coefficient of x and the product of the roots is given by the constant term. Let R and r be the bigger and smaller root of the first equation, then
R + r = 7 9 R r = 1
The second equation with smaller root ρ gives
R + ρ = b R ρ = 1
Using these pairs of equations to eliminate the smaller roots gives
7 9 = R + R 1 … ( 1 ) b = R + R 1
Squaring this last equation gives
b 2 = R + R 1 + 2
Then using equation (1) gives
b 2 = 7 9 + 2 = 8 1
And so b (we are told t is positive) is 9
Gentle reminder that Desmos exists
Got the correct answer, but did it the brute force way.
I only wanted to comment that you may want to be more precise with your definitions. Specifically, x 2 − a x + 1 = 0 has real solutions only when ∣ a ∣ ≥ 2 ; the argument is the same when you replace a with b .
Aside from that, nice problem!
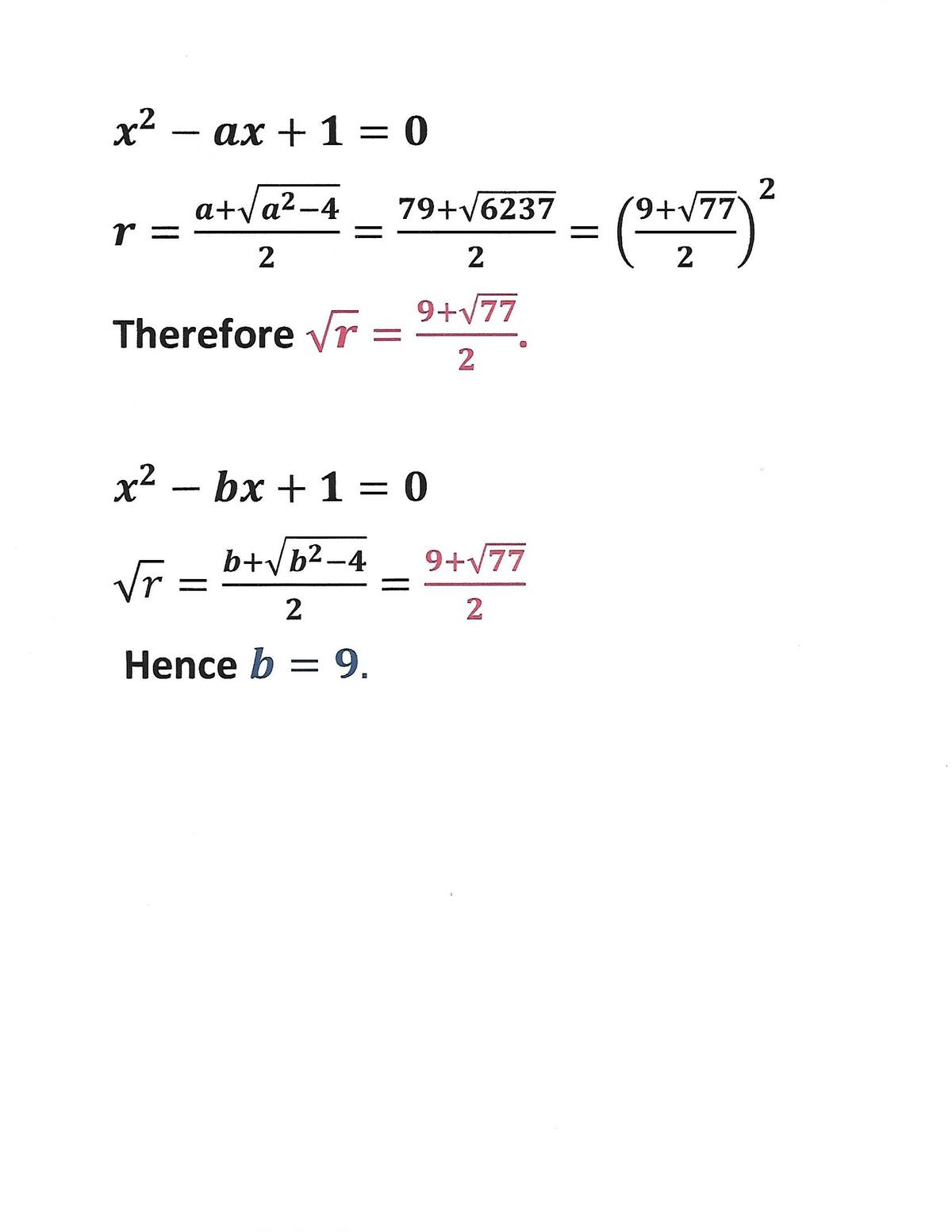
Instead of directly using x 2 − 7 9 x + 1 = 0 , we will simply use x 2 − a x + 1 = 0 as a generalization (assuming a > 0 of course), and then obtain a formula we can just plug in.
We present two solutions:
Solution 1: r 2 − a r + 1 = 0 a = r r 2 + 1 r − b r + 1 = 0 b = r r + 1 b 2 = r r 2 + 2 r + 1 b 2 = a + 2 b = a + 2
Solution 2: ( x 2 − b x + 1 ) ( x 2 + b x + 1 ) = x 4 − ( b 2 − 2 ) x 2 + 1 = 0 b 2 − 2 = a b = a + 2
Plugging in our value of a , we get that b = 7 9 + 2 = 9 .