The Secants....

Geometry Level 2

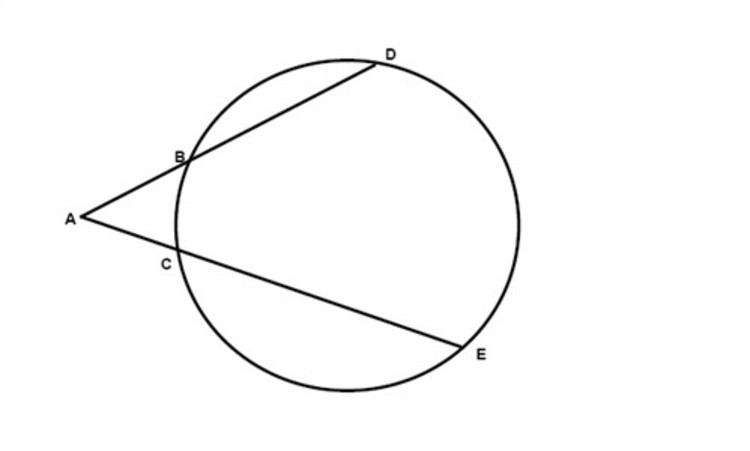Two secants are drawn to a circle from the same external point. The segments of one secant that lie inside and outside the circle are 9 cm and 3 cm, respectively. The segment of the other secant that lies inside the circle is twice its segment lying outside the circle. What is the length (in cm) of the second secant?

6\sqrt{3} 5\sqrt{2} 8\sqrt{3} 7\sqrt{2}

Sittie Ainah Suma by Sittie Ainah Suma , 28, Philippines

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

We have A B = 3 AB=3 , B D = 9 BD=9 , A C = x AC=x and C E = 2 x CE=2x . Then, by Power of a Point we have: A B ( A B + B D ) = A C ( A C + C E ) AB(AB+BD)=AC(AC+CE) . That is: 3 ( 3 + 9 ) = x ( x + 2 x ) 3(3+9)=x(x+2x) . Solving that, we obtain:

36 = 3 x 2 ⟹ x = 2 3 36=3x^2 \Longrightarrow x=2\sqrt{3}

Finally, the length of A E AE is 3 x 3x , so A E = 6 3 AE=6\sqrt{3} .

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...