The square

Geometry Level pending

The figure shows a square ABCD , the point F divides the length of DC such that DF : FC = ( n - 1 ) : ( n + 1 ) , where as the point E divides the length of AB such that AE : EB = ( n+ 1 ) : ( n - 1 ) . If the percentage of the shaded area to the area of the square ABCD = 24 % . find the value of n ?


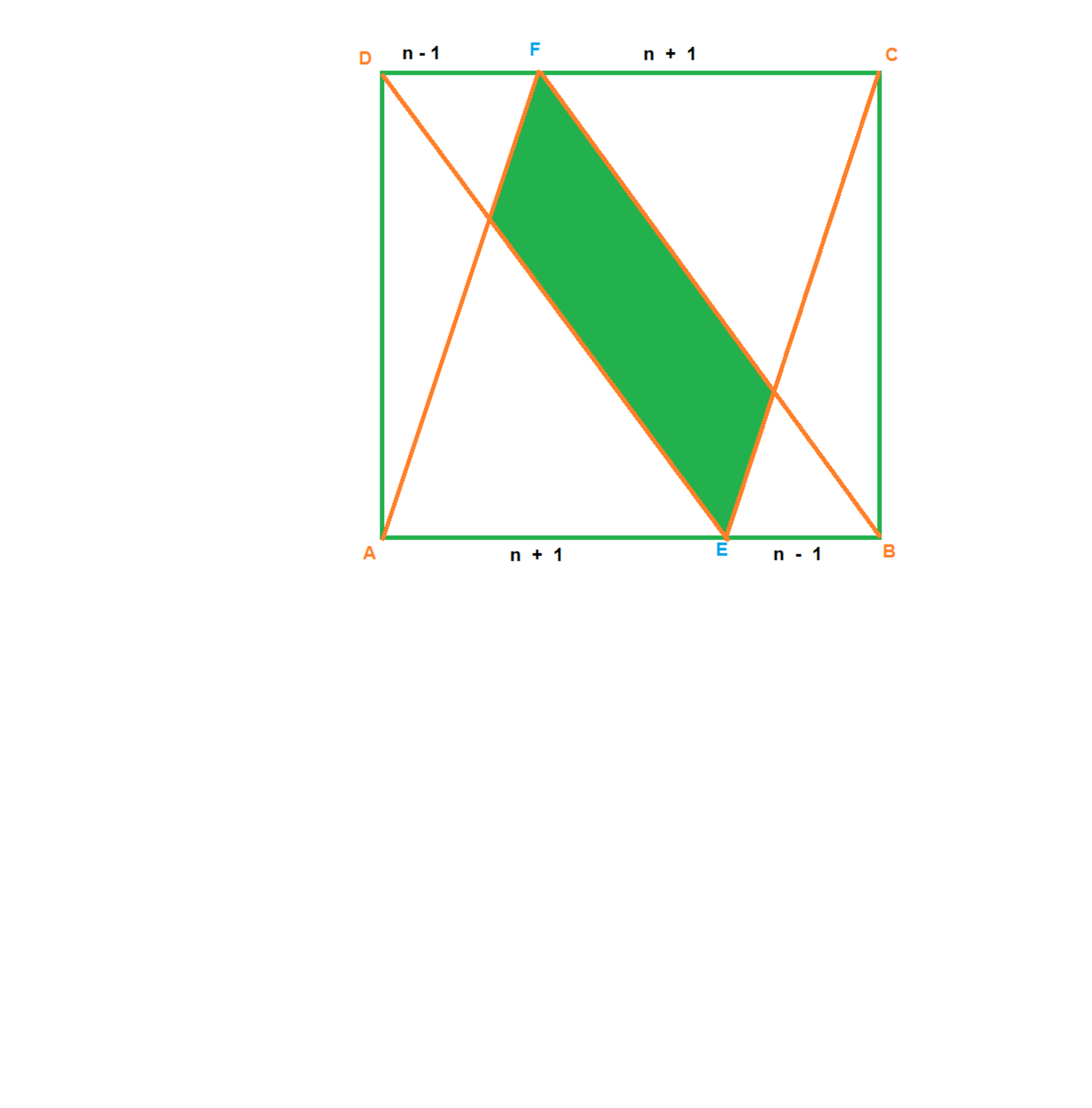
The answer is 5.

Aziz Alasha by Aziz Alasha , 59, Sudan

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Tom Engelsman
May 13, 2021

If we place square A B C D ABCD of side length 2 n 2n in the first quadrant of the x y − xy- plane such that:

1) The green parallelogram's other vertices are E ′ E' and F ′ ; F';

2) A = ( 0 , 0 ) A = (0,0) ;

3) △ A D F = △ B C E = 1 2 ( 2 n ) ( n − 1 ) ; \triangle{ADF} = \triangle{BCE} = \frac{1}{2}(2n)(n-1);

4) △ A F ′ E = △ C F E ′ = 1 2 ( n + 1 ) ( n + 1 ) ; \triangle{AF'E} = \triangle{CFE'} = \frac{1}{2}(n+1)(n+1);

then we have:

( 2 n ) 2 − [ 2 ( 1 / 2 ) ( 2 n ) ( n − 1 ) + 2 ( 1 / 2 ) ( n + 1 ) 2 ] ( 2 n ) 2 = 0.24 ; \Large \frac{(2n)^2 - [2(1/2)(2n)(n-1) + 2(1/2)(n+1)^{2}]}{(2n)^2} = 0.24;

or 4 n 2 − ( 3 n 2 + 1 ) 4 n 2 = 0.24 ; \Large \frac{4n^2 - (3n^2+1)}{4n^2} = 0.24;

or ( 1 − 0.96 ) n 2 = 1 ; (1-0.96)n^2 = 1;

or n = 5 . \boxed{n=5}.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

1 pending report

Vote up reports you agree with

×

Problem Loading...

Note Loading...

Set Loading...